Goodness-of-fit Test for Latent Block Models
Paper and Code
Jul 09, 2019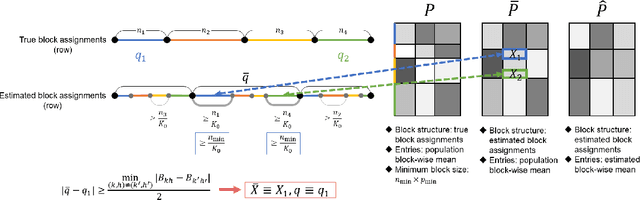
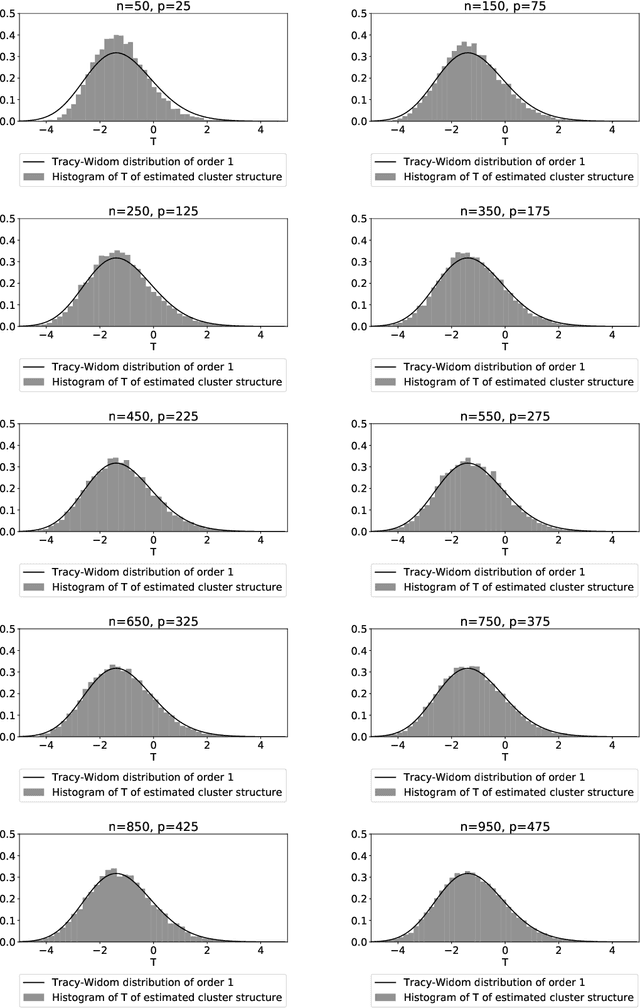
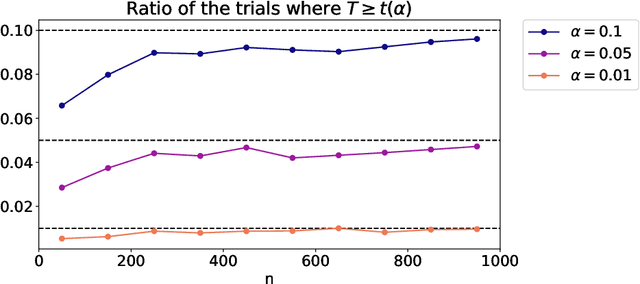
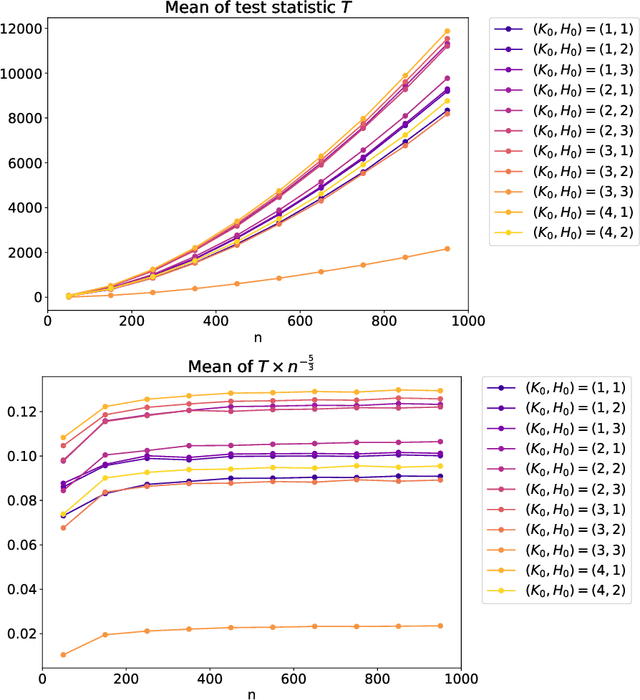
Latent block models are used for probabilistic biclustering, which is shown to be an effective method for analyzing various relational data sets. However, there has been no statistical test method for determining the row and column cluster numbers of latent block models. Recent studies have constructed statistical-test-based methods for stochastic block models, which assume that the observed matrix is a square symmetric matrix and that the cluster assignments are the same for rows and columns. In this study, we developed a new goodness-of-fit test for latent block models to test whether an observed data matrix fits a given set of row and column cluster numbers, or it consists of more clusters in at least one direction of the row and the column. To construct the test method, we used a result from the random matrix theory for a sample covariance matrix. We experimentally demonstrated the effectiveness of the proposed method by showing the asymptotic behavior of the test statistic and measuring the test accuracy.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge