Global exponential stability of primal-dual gradient flow dynamics based on the proximal augmented Lagrangian: A Lyapunov-based approach
Paper and Code
Oct 02, 2019
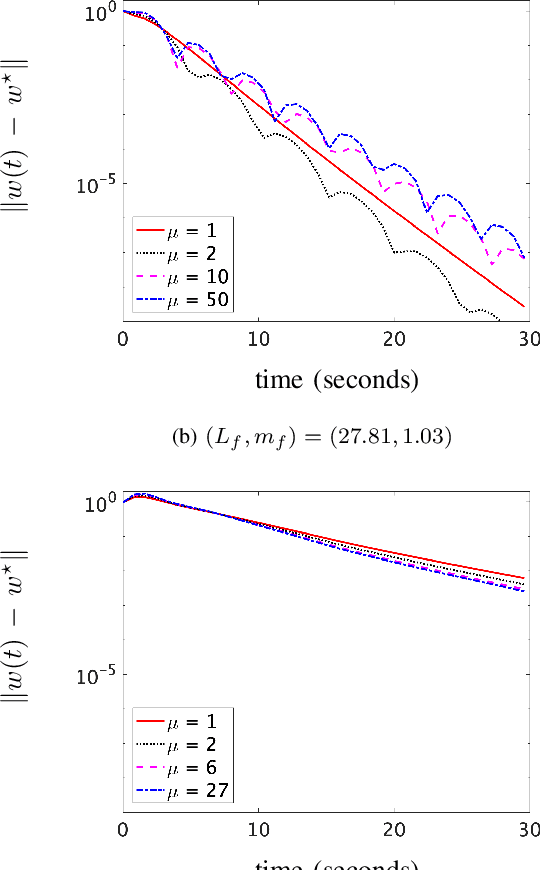
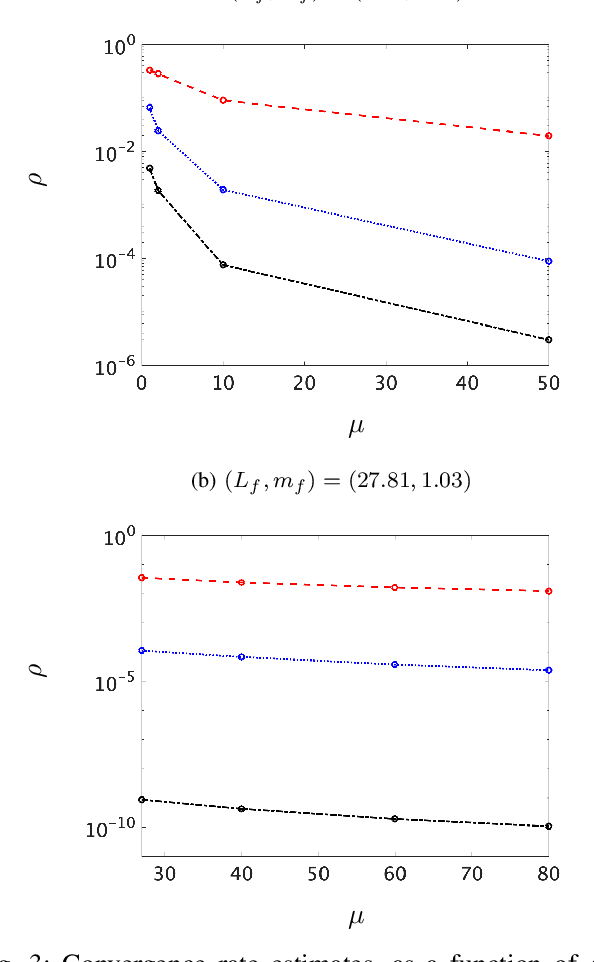
For a class of nonsmooth composite optimization problems with linear equality constraints, we utilize a Lyapunov-based approach to establish the global exponential stability of the primal-dual gradient flow dynamics based on the proximal augmented Lagrangian. The result holds when the differentiable part of the objective function is strongly convex with a Lipschitz continuous gradient; the non-differentiable part is proper, lower semi-continuous, and convex; and the matrix in the linear constraint is full row rank. Our quadratic Lyapunov function generalizes recent result from strongly convex problems with either affine equality or inequality constraints to a broader class of composite optimization problems with nonsmooth regularizers and it provides a worst-case lower bound of the exponential decay rate. Finally, we use computational experiments to demonstrate that our convergence rate estimate is less conservative than the existing alternatives.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge