Generalized Visual Information Analysis via Tensorial Algebra

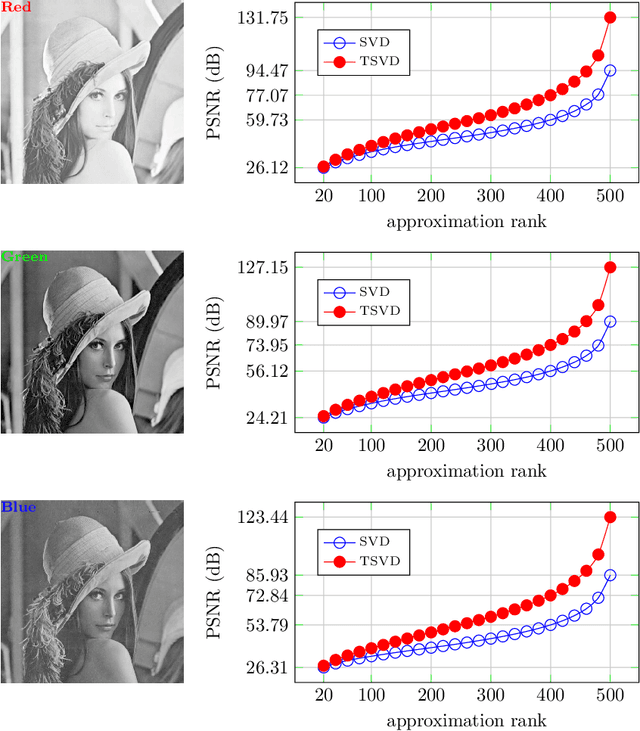
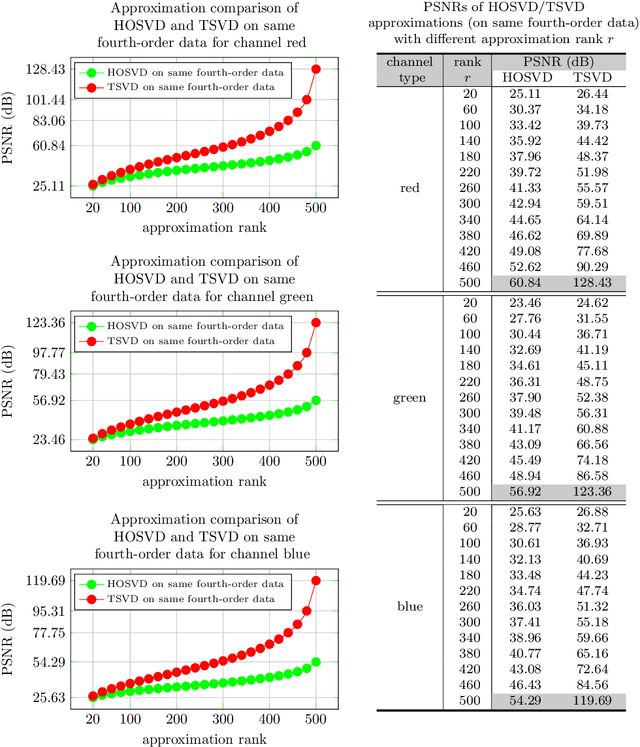
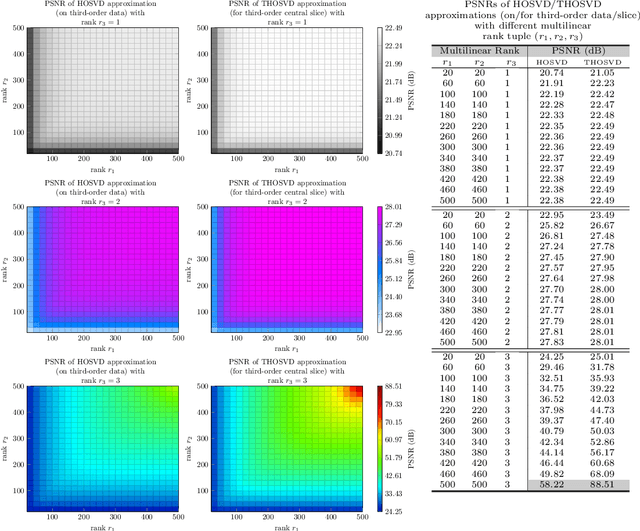
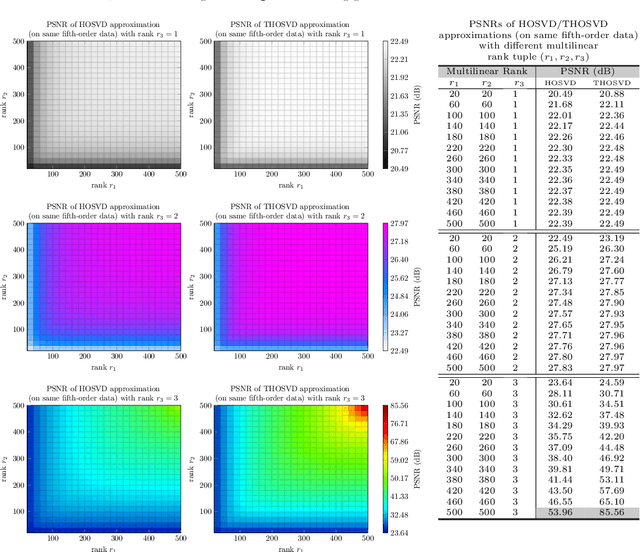
High order data is modeled using matrices whose entries are numerical arrays of a fixed size. These arrays, called t-scalars, form a commutative ring under the convolution product. Matrices with elements in the ring of t-scalars are referred to as t-matrices. The t-matrices can be scaled, added and multiplied in the usual way. There are t-matrix generalizations of positive matrices, orthogonal matrices and Hermitian symmetric matrices. With the t-matrix model, it is possible to generalize many well-known matrix algorithms. In particular, the t-matrices are used to generalize the SVD (Singular Value Decomposition), HOSVD (High Order SVD), PCA (Principal Component Analysis), 2DPCA (Two Dimensional PCA) and GCA (Grassmannian Component Analysis). The generalized t-matrix algorithms, namely TSVD, THOSVD,TPCA, T2DPCA and TGCA, are applied to low-rank approximation, reconstruction,and supervised classification of images. Experiments show that the t-matrix algorithms compare favorably with standard matrix algorithms.