Gaussian Processes for Missing Value Imputation
Paper and Code
Apr 10, 2022

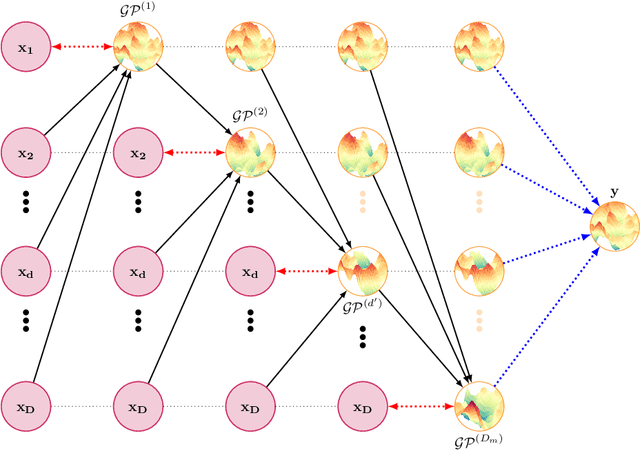

Missing values are common in many real-life datasets. However, most of the current machine learning methods can not handle missing values. This means that they should be imputed beforehand. Gaussian Processes (GPs) are non-parametric models with accurate uncertainty estimates that combined with sparse approximations and stochastic variational inference scale to large data sets. Sparse GPs can be used to compute a predictive distribution for missing data. Here, we present a hierarchical composition of sparse GPs that is used to predict missing values at each dimension using all the variables from the other dimensions. We call the approach missing GP (MGP). MGP can be trained simultaneously to impute all observed missing values. Specifically, it outputs a predictive distribution for each missing value that is then used in the imputation of other missing values. We evaluate MGP in one private clinical data set and four UCI datasets with a different percentage of missing values. We compare the performance of MGP with other state-of-the-art methods for imputing missing values, including variants based on sparse GPs and deep GPs. The results obtained show a significantly better performance of MGP.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge