From-Below Approximations in Boolean Matrix Factorization: Geometry and New Algorithm
Paper and Code
Jun 20, 2013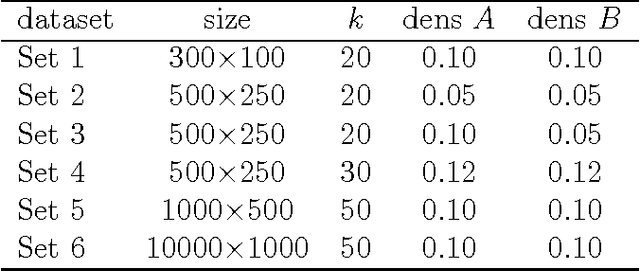
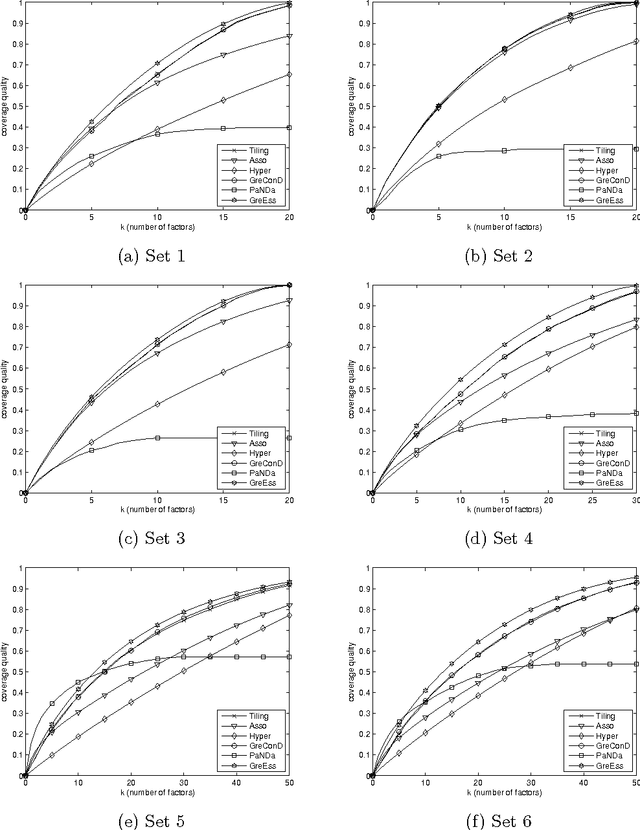
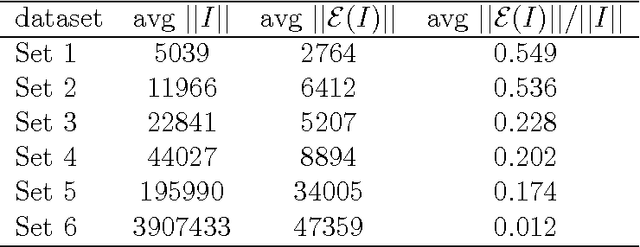
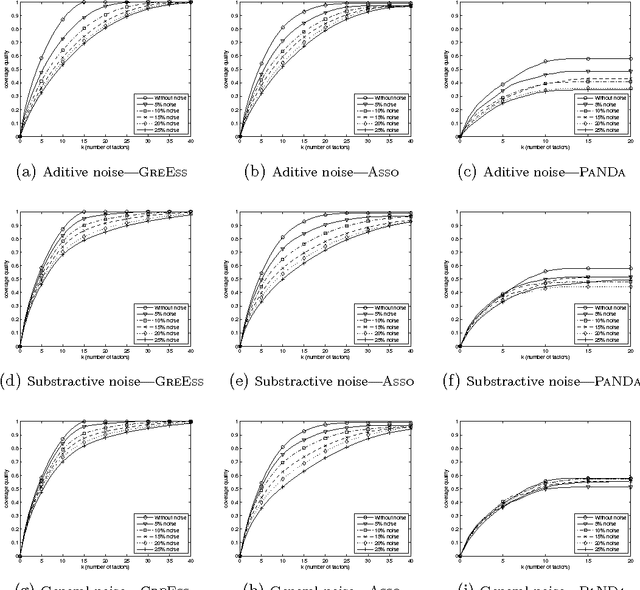
We present new results on Boolean matrix factorization and a new algorithm based on these results. The results emphasize the significance of factorizations that provide from-below approximations of the input matrix. While the previously proposed algorithms do not consider the possibly different significance of different matrix entries, our results help measure such significance and suggest where to focus when computing factors. An experimental evaluation of the new algorithm on both synthetic and real data demonstrates its good performance in terms of good coverage by the first k factors as well as a small number of factors needed for exact decomposition and indicates that the algorithm outperforms the available ones in these terms. We also propose future research topics.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge