Explicitly Bayesian Regularizations in Deep Learning
Paper and Code
Oct 22, 2019

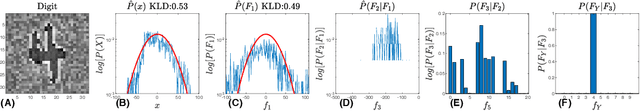
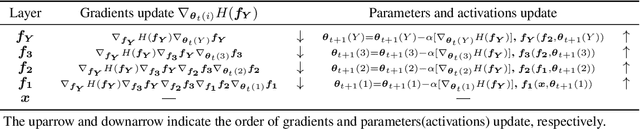
Generalization is essential for deep learning. In contrast to previous works claiming that Deep Neural Networks (DNNs) have an implicit regularization implemented by the stochastic gradient descent, we demonstrate explicitly Bayesian regularizations in a specific category of DNNs, i.e., Convolutional Neural Networks (CNNs). First, we introduce a novel probabilistic representation for the hidden layers of CNNs and demonstrate that CNNs correspond to Bayesian networks with the serial connection. Furthermore, we show that the hidden layers close to the input formulate prior distributions, thus CNNs have explicitly Bayesian regularizations based on the Bayesian regularization theory. In addition, we clarify two recently observed empirical phenomena that are inconsistent with traditional theories of generalization. Finally, we validate the proposed theory on a synthetic dataset
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge