Échantillonnage de signaux sur graphes via des processus déterminantaux
Paper and Code
Jul 05, 2017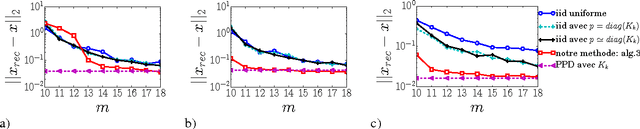
We consider the problem of sampling k-bandlimited graph signals, ie, linear combinations of the first k graph Fourier modes. We know that a set of k nodes embedding all k-bandlimited signals always exists, thereby enabling their perfect reconstruction after sampling. Unfortunately, to exhibit such a set, one needs to partially diagonalize the graph Laplacian, which becomes prohibitive at large scale. We propose a novel strategy based on determinantal point processes that side-steps partial diagonalisation and enables reconstruction with only O(k) samples. While doing so, we exhibit a new general algorithm to sample determinantal process, faster than the state-of-the-art algorithm by an order k.
* GRETSI, Sep 2017, Juan-les-Pins, France * in French
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge