Dynamic Optimization Fabrics for Motion Generation
Paper and Code
May 17, 2022
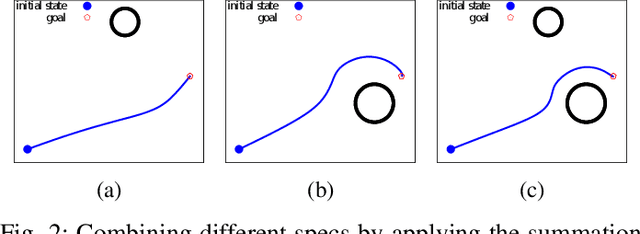
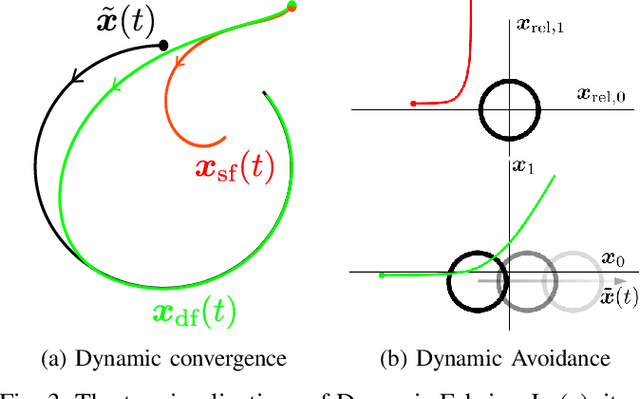
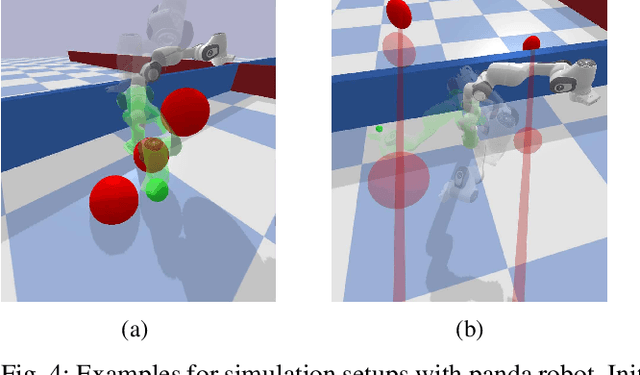
Optimization fabrics represent a geometric approach to real-time motion planning, where trajectories are designed by the composition of several differential equations that exhibit a desired motion behavior. We generalize this framework to dynamic scenarios and prove that fundamental properties can be conserved. We show that convergence to trajectories and avoidance of moving obstacles can be guaranteed using simple construction rules of the components. Additionally, we present the first quantitative comparisons between optimization fabrics and model predictive control and show that optimization fabrics can generate similar trajectories with better scalability, and thus, much higher replanning frequency (up to 500 Hz with a 7 degrees of freedom robotic arm). Finally, we present empirical results on several robots, including a non-holonomic mobile manipulator with 10 degrees of freedom, supporting the theoretical findings.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge