Deep Network Approximation with Discrepancy Being Reciprocal of Width to Power of Depth

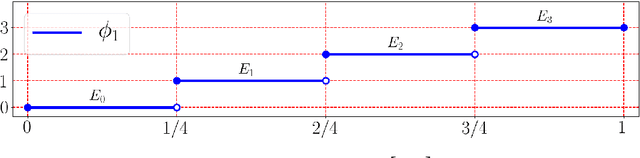
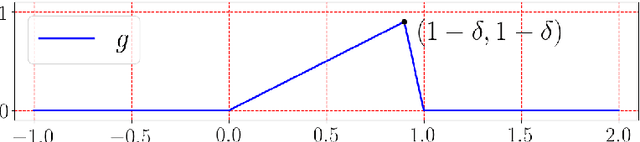
A new network with super approximation power is introduced. This network is built with Floor ($\lfloor x\rfloor$) and ReLU ($\max\{0,x\}$) activation functions and hence we call such networks as Floor-ReLU networks. It is shown by construction that Floor-ReLU networks with width $\max\{d,\, 5N+13\}$ and depth $64dL+3$ can pointwise approximate a Lipschitz continuous function $f$ on $[0,1]^d$ with an exponential approximation rate $3\mu\sqrt{d}\,N^{-\sqrt{L}}$, where $\mu$ is the Lipschitz constant of $f$. More generally for an arbitrary continuous function $f$ on $[0,1]^d$ with a modulus of continuity $\omega_f(\cdot)$, the constructive approximation rate is $\omega_f(\sqrt{d}\,N^{-\sqrt{L}})+2\omega_f(\sqrt{d}){N^{-\sqrt{L}}}$. As a consequence, this new network overcomes the curse of dimensionality in approximation power since this approximation order is essentially $\sqrt{d}$ times a function of $N$ and $L$ independent of $d$.