Convergence to the fixed-node limit in deep variational Monte Carlo

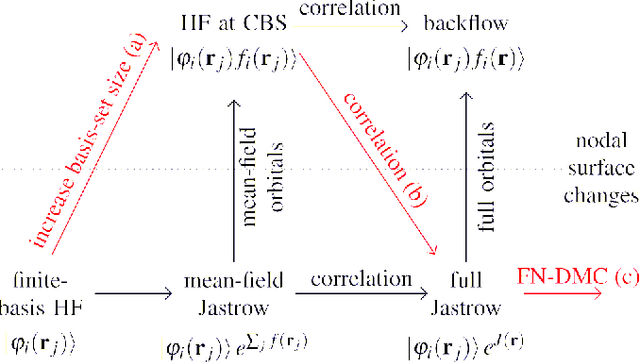
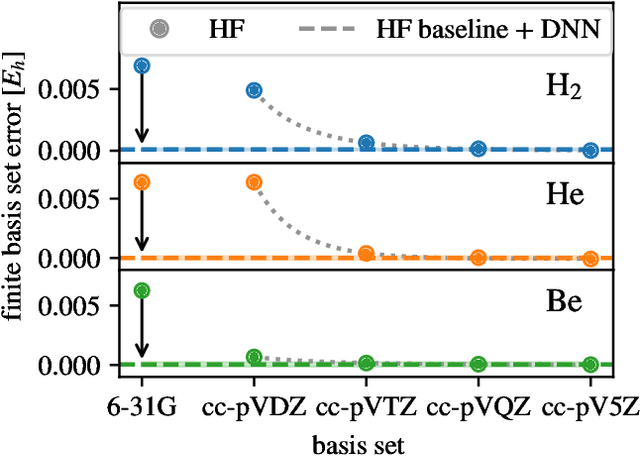
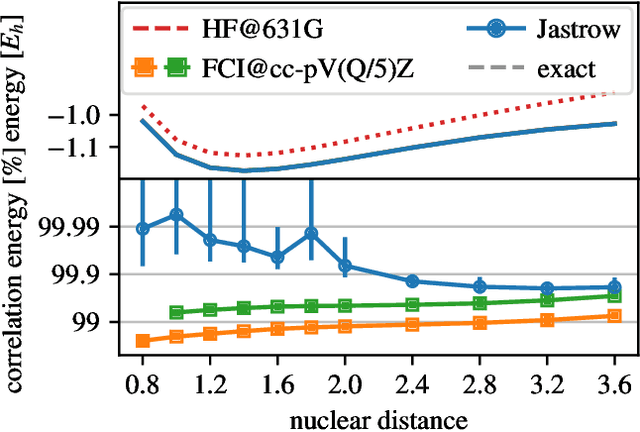
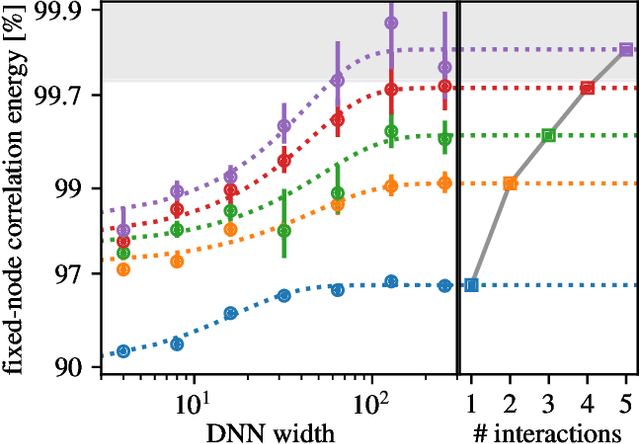
Variational quantum Monte Carlo (QMC) is an ab-initio method for solving the electronic Schr\"odinger equation that is exact in principle, but limited by the flexibility of the available ansatzes in practice. The recently introduced deep QMC approach, specifically two deep-neural-network ansatzes PauliNet and FermiNet, allows variational QMC to reach the accuracy of diffusion QMC, but little is understood about the convergence behavior of such ansatzes. Here, we analyze how deep variational QMC approaches the fixed-node limit with increasing network size. First, we demonstrate that a deep neural network can overcome the limitations of a small basis set and reach the mean-field complete-basis-set limit. Moving to electron correlation, we then perform an extensive hyperparameter scan of a deep Jastrow factor for LiH and H$_4$ and find that variational energies at the fixed-node limit can be obtained with a sufficiently large network. Finally, we benchmark mean-field and many-body ansatzes on H$_2$O, increasing the fraction of recovered fixed-node correlation energy by half an order of magnitude compared to previous VMC results. This analysis helps understanding the superb performance of deep variational ansatzes, and will guide future improvements of the neural network architectures in deep QMC.