Complex Amplitude-Phase Boltzmann Machines
Paper and Code
May 04, 2020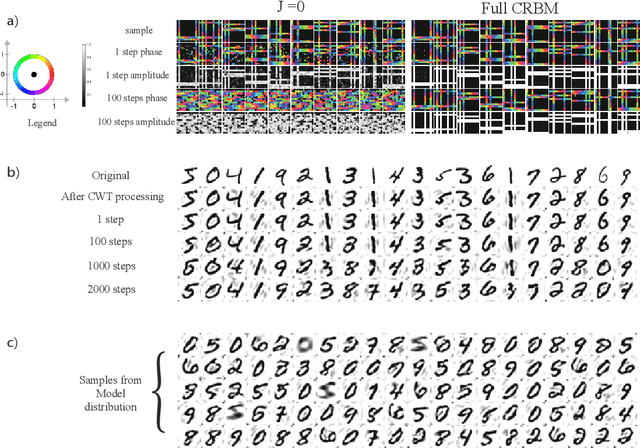
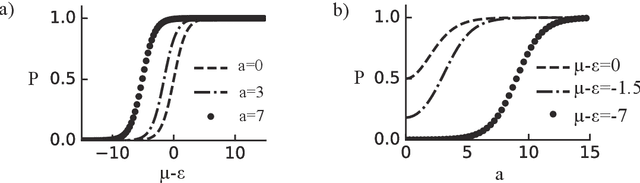
We extend the framework of Boltzmann machines to a network of complex-valued neurons with variable amplitudes, referred to as Complex Amplitude-Phase Boltzmann machine (CAP-BM). The model is capable of performing unsupervised learning on the amplitude and relative phase distribution in complex data. The sampling rule of the Gibbs distribution and the learning rules of the model are presented. Learning in a Complex Amplitude-Phase restricted Boltzmann machine (CAP-RBM) is demonstrated on synthetic complex-valued images, and handwritten MNIST digits transformed by a complex wavelet transform. Specifically, we show the necessity of a new amplitude-amplitude coupling term in our model. The proposed model is potentially valuable for machine learning tasks involving complex-valued data with amplitude variation, and for developing algorithms for novel computation hardware, such as coupled oscillators and neuromorphic hardware, on which Boltzmann sampling can be executed in the complex domain.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge