Batch Bayesian Optimization via Particle Gradient Flows
Paper and Code
Sep 10, 2022
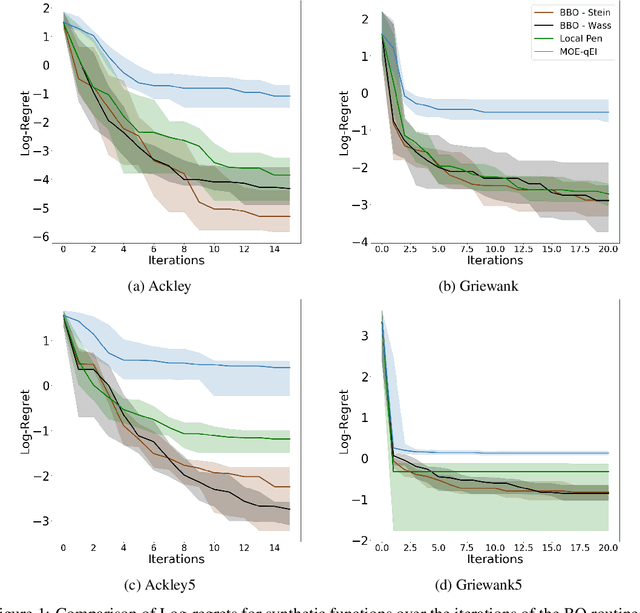
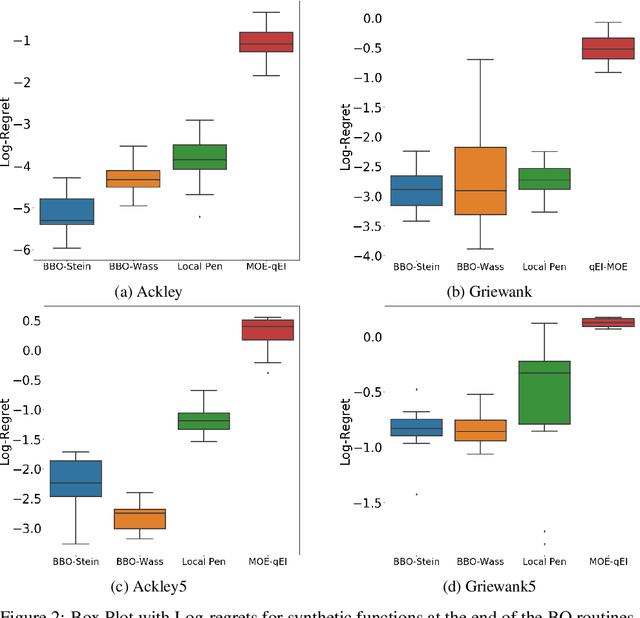
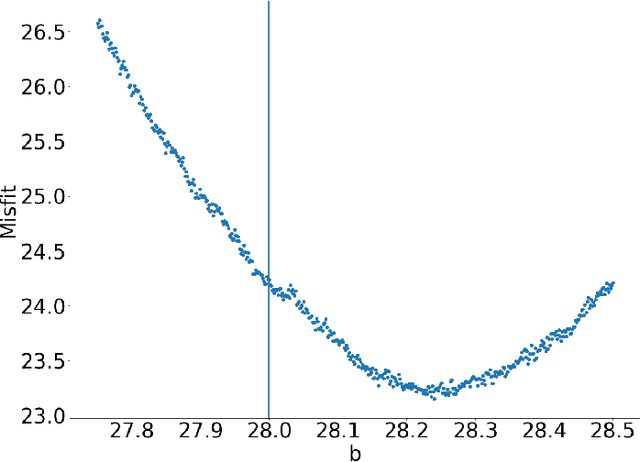
Bayesian Optimisation (BO) methods seek to find global optima of objective functions which are only available as a black-box or are expensive to evaluate. Such methods construct a surrogate model for the objective function, quantifying the uncertainty in that surrogate through Bayesian inference. Objective evaluations are sequentially determined by maximising an acquisition function at each step. However, this ancilliary optimisation problem can be highly non-trivial to solve, due to the non-convexity of the acquisition function, particularly in the case of batch Bayesian optimisation, where multiple points are selected in every step. In this work we reformulate batch BO as an optimisation problem over the space of probability measures. We construct a new acquisition function based on multipoint expected improvement which is convex over the space of probability measures. Practical schemes for solving this `inner' optimisation problem arise naturally as gradient flows of this objective function. We demonstrate the efficacy of this new method on different benchmark functions and compare with state-of-the-art batch BO methods.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge