An Application of a Multivariate Estimation of Distribution Algorithm to Cancer Chemotherapy
Paper and Code
May 17, 2022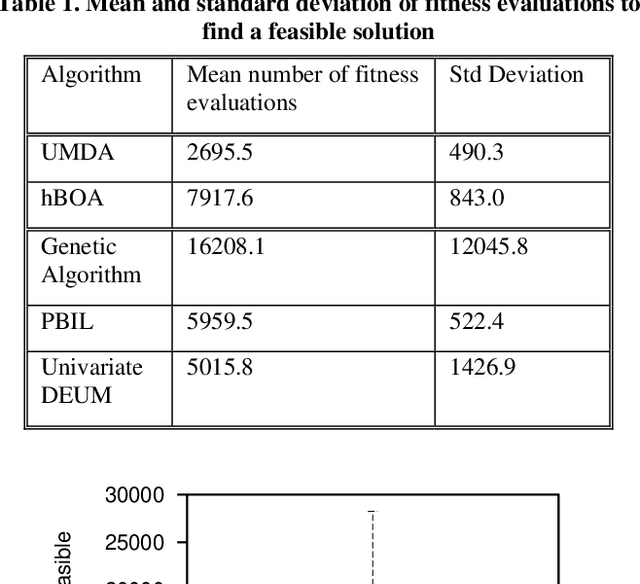
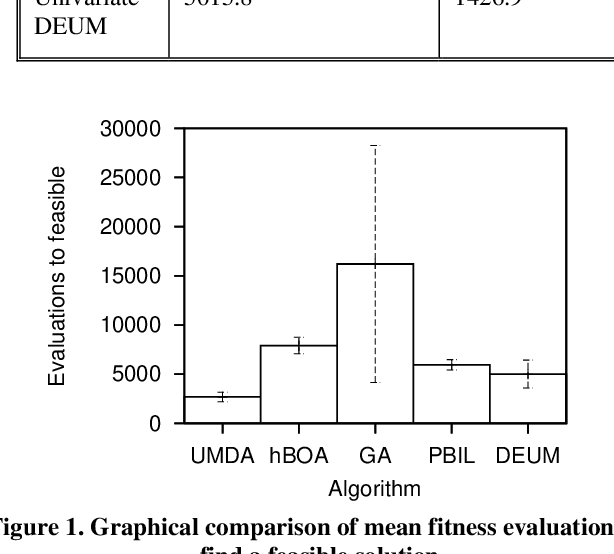
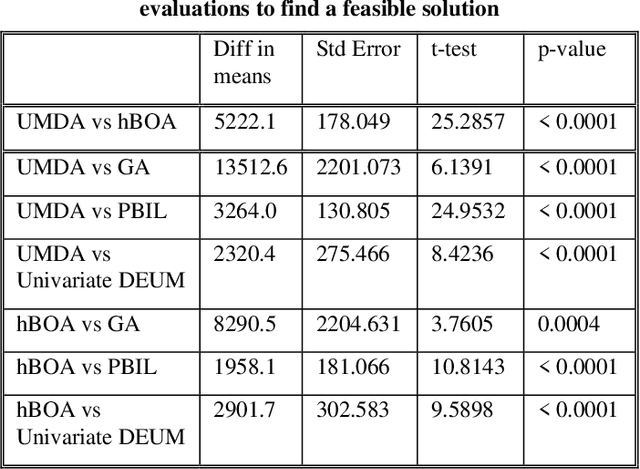
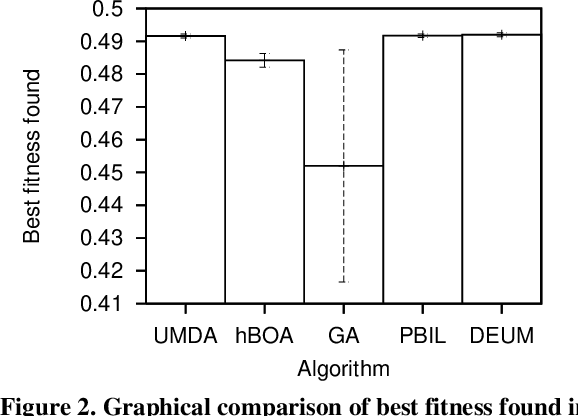
Chemotherapy treatment for cancer is a complex optimisation problem with a large number of interacting variables and constraints. A number of different probabilistic algorithms have been applied to it with varying success. In this paper we expand on this by applying two estimation of distribution algorithms to the problem. One is UMDA, which uses a univariate probabilistic model similar to previously applied EDAs. The other is hBOA, the first EDA using a multivariate probabilistic model to be applied to the chemotherapy problem. While instinct would lead us to predict that the more sophisticated algorithm would yield better performance on a complex problem like this, we show that it is outperformed by the algorithms using the simpler univariate model. We hypothesise that this is caused by the more sophisticated algorithm being impeded by the large number of interactions in the problem which are unnecessary for its solution.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge