Algebraic and Statistical Properties of the Ordinary Least Squares Interpolator
Paper and Code
Sep 27, 2023
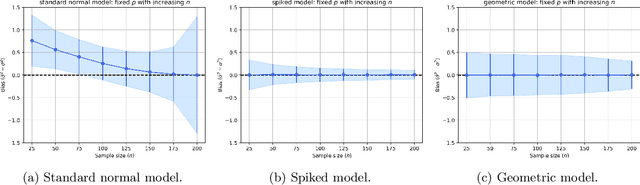

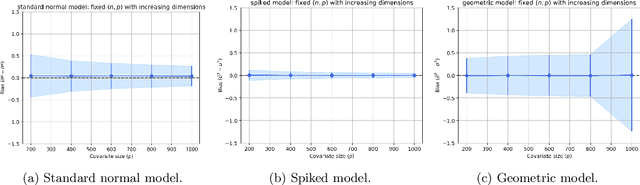
Deep learning research has uncovered the phenomenon of benign overfitting for over-parameterized statistical models, which has drawn significant theoretical interest in recent years. Given its simplicity and practicality, the ordinary least squares (OLS) interpolator has become essential to gain foundational insights into this phenomenon. While properties of OLS are well established in classical settings, its behavior in high-dimensional settings is less explored (unlike for ridge or lasso regression) though significant progress has been made of late. We contribute to this growing literature by providing fundamental algebraic and statistical results for the minimum $\ell_2$-norm OLS interpolator. In particular, we provide high-dimensional algebraic equivalents of (i) the leave-$k$-out residual formula, (ii) Cochran's formula, and (iii) the Frisch-Waugh-Lovell theorem. These results aid in understanding the OLS interpolator's ability to generalize and have substantive implications for causal inference. Additionally, under the Gauss-Markov model, we present statistical results such as a high-dimensional extension of the Gauss-Markov theorem and an analysis of variance estimation under homoskedastic errors. To substantiate our theoretical contributions, we conduct simulation studies that further explore the stochastic properties of the OLS interpolator.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge