AI-Lorenz: A physics-data-driven framework for black-box and gray-box identification of chaotic systems with symbolic regression
Paper and Code
Dec 21, 2023
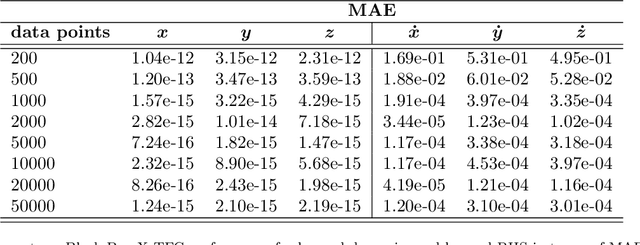
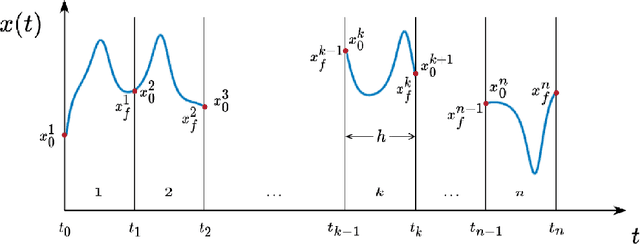
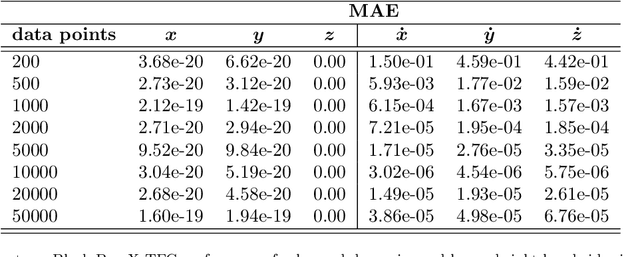
Discovering mathematical models that characterize the observed behavior of dynamical systems remains a major challenge, especially for systems in a chaotic regime. The challenge is even greater when the physics underlying such systems is not yet understood, and scientific inquiry must solely rely on empirical data. Driven by the need to fill this gap, we develop a framework that learns mathematical expressions modeling complex dynamical behaviors by identifying differential equations from noisy and sparse observable data. We train a small neural network to learn the dynamics of a system, its rate of change in time, and missing model terms, which are used as input for a symbolic regression algorithm to autonomously distill the explicit mathematical terms. This, in turn, enables us to predict the future evolution of the dynamical behavior. The performance of this framework is validated by recovering the right-hand sides and unknown terms of certain complex, chaotic systems such as the well-known Lorenz system, a six-dimensional hyperchaotic system, and the non-autonomous Sprott chaotic system, and comparing them with their known analytical expressions.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge