Adiabatic Quantum Optimization Fails to Solve the Knapsack Problem
Paper and Code
Aug 17, 2020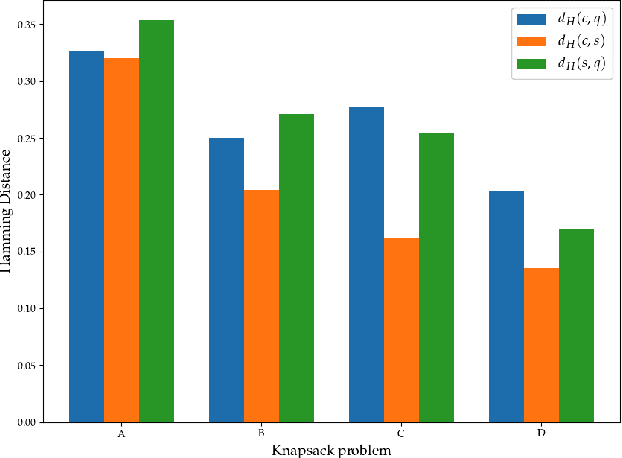
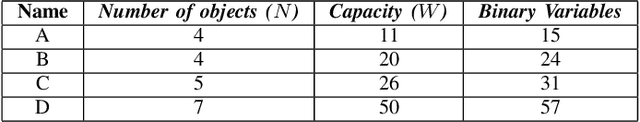
In this work, we attempt to solve the integer-weight knapsack problem using the D-Wave 2000Q adiabatic quantum computer. The knapsack problem is a well-known NP-complete problem in computer science, with applications in economics, business, finance, etc. We attempt to solve a number of small knapsack problems whose optimal solutions are known; we find that adiabatic quantum optimization fails to produce solutions corresponding to optimal filling of the knapsack in all problem instances. We compare results obtained on the quantum hardware to the classical simulated annealing algorithm and two solvers employing a hybrid branch-and-bound algorithm. The simulated annealing algorithm also fails to produce the optimal filling of the knapsack, though solutions obtained by simulated and quantum annealing are no more similar to each other than to the correct solution. We discuss potential causes for this observed failure of adiabatic quantum optimization.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge