A Universal Quantum Computer From Relativistic Motion
Paper and Code
Oct 31, 2024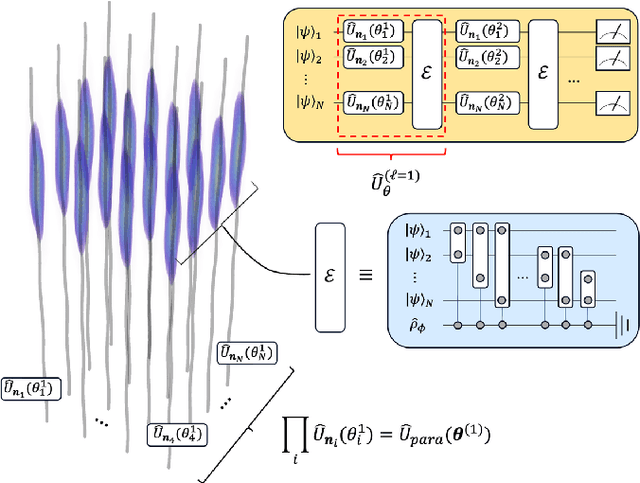

We present an explicit construction of a relativistic quantum computing architecture using a variational quantum circuit approach that is shown to allow for universal quantum computing. The variational quantum circuit consists of tunable single-qubit rotations and entangling gates that are implemented successively. The single qubit rotations are parameterized by the proper time intervals of the qubits' trajectories and can be tuned by varying their relativistic motion in spacetime. The entangling layer is mediated by a relativistic quantum field instead of through direct coupling between the qubits. Within this setting, we give a prescription for how to use quantum field-mediated entanglement and manipulation of the relativistic motion of qubits to obtain a universal gate set, for which compact non-perturbative expressions that are valid for general spacetimes are also obtained. We also derive a lower bound on the channel fidelity that shows the existence of parameter regimes in which all entangling operations are effectively unitary, despite the noise generated from the presence of a mediating quantum field. Finally, we consider an explicit implementation of the quantum Fourier transform with relativistic qubits.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge