A Technique for Deriving Equational Conditions on the Denavit-Hartenberg Parameters of 6R Linkages that are Necessary for Movability
Paper and Code
Aug 25, 2015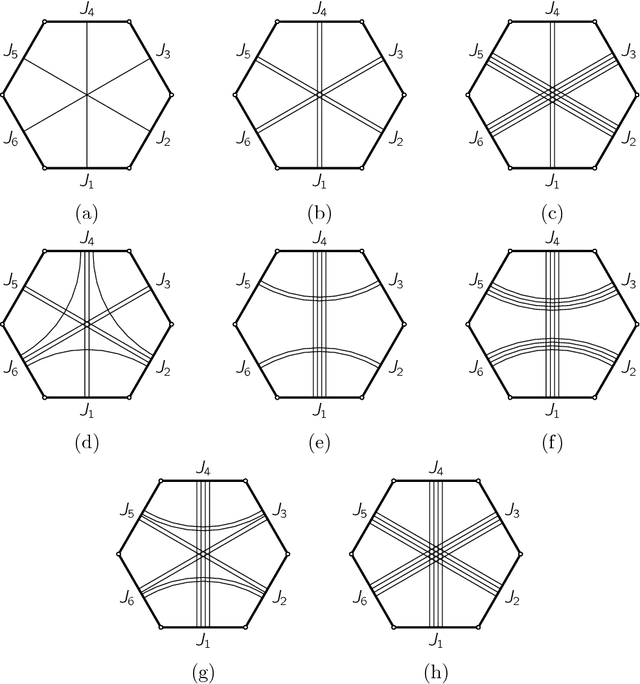
A closed 6R linkage is generically rigid. Special cases may be mobile. Many families of mobile 6R linkages have been characterised in terms of the invariant Denavit-Hartenberg parameters of the linkage. In other words, many sufficient conditions for mobility are known. In this paper we give, for the first time, equational conditions on the invariant Denavit-Hartenberg parameters that are necessary for mobility. The method is based on the theory of bonds. We illustrate the method by deriving the equational conditions for various well-known linkages (Bricard's line symmetric linkage, Hooke's linkage, Dietmaier's linkage, and recent a generalization of Bricard's orthogonal linkage), starting from their bond diagrams; and by deriving the equations for another bond diagram, thereby discovering a new mobile 6R linkage.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge