A Novel Approach to Sparse Inverse Covariance Estimation Using Transform Domain Updates and Exponentially Adaptive Thresholding
Paper and Code
Nov 16, 2018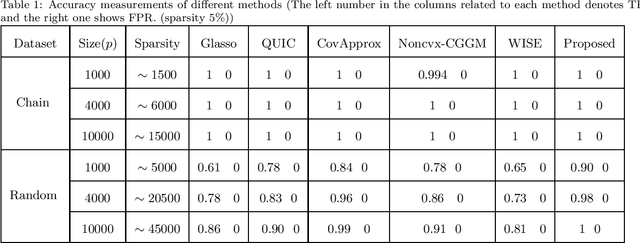
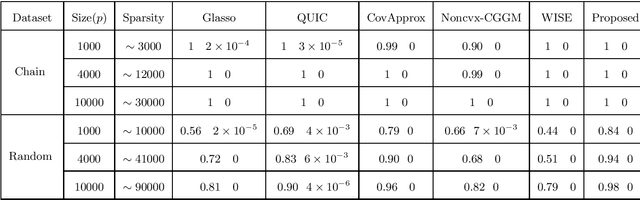
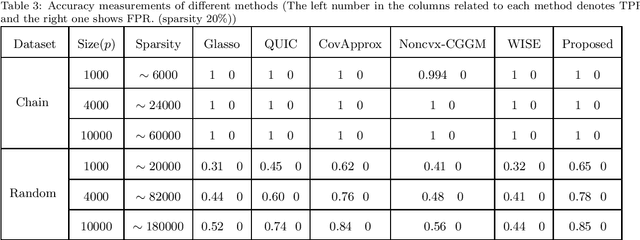
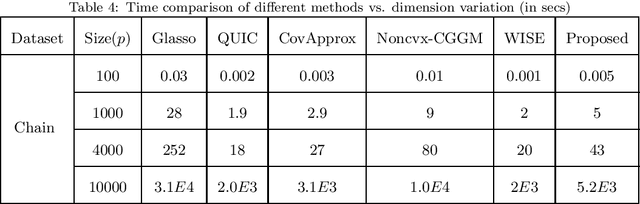
Sparse Inverse Covariance Estimation (SICE) is useful in many practical data analyses. Recovering the connectivity, non-connectivity graph of covariates is classified amongst the most important data mining and learning problems. In this paper, we introduce a novel SICE approach using adaptive thresholding. Our method is based on updates in a transformed domain of the desired matrix and exponentially decaying adaptive thresholding in the main domain (Inverse Covariance matrix domain). In addition to the proposed algorithm, the convergence analysis is also provided. In the Numerical Experiments Section, we show that the proposed method outperforms state-of-the-art methods in terms of accuracy.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge