A Convexly Constrained LiGME Model and Its Proximal Splitting Algorithm
Paper and Code
May 14, 2021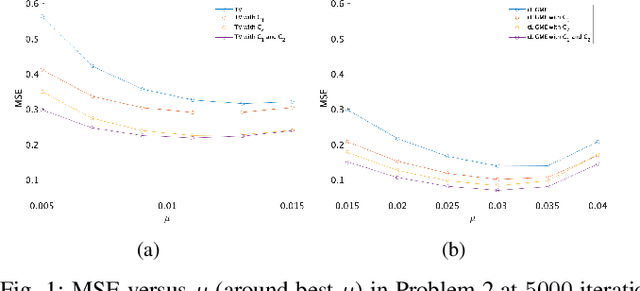

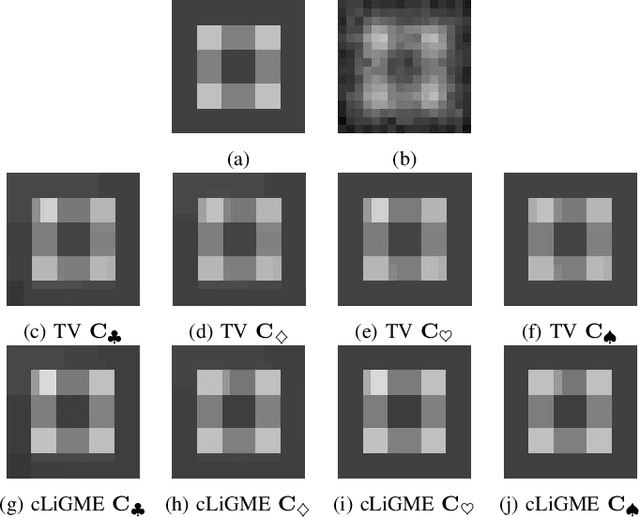
For the sparsity-rank-aware least squares estimations, the LiGME (Linearly involved Generalized Moreau Enhanced) model was established recently in [Abe, Yamagishi, Yamada, 2020] to use certain nonconvex enhancements of linearly involved convex regularizers without losing their overall convexities. In this paper, for further advancement of the LiGME model by incorporating multiple a priori knowledge as hard convex constraints, we newly propose a convexly constrained LiGME (cLiGME) model. The cLiGME model can utilize multiple convex constraints while preserving benefits achieved by the LiGME model. We also present a proximal splitting type algorithm for the proposed cLiGME model. Numerical experiments demonstrate the efficacy of the proposed model and the proposed optimization algorithm in a scenario of signal processing application.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge