Basis Function Based Data Driven Learning for the Inverse Problem of Electrocardiography

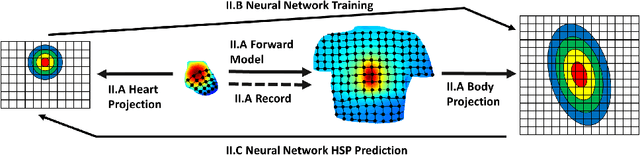
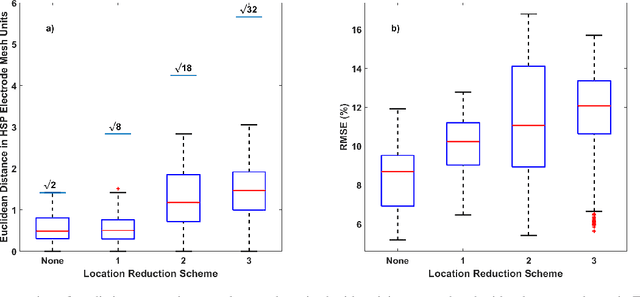
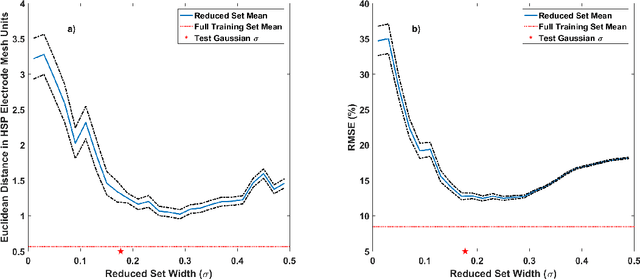
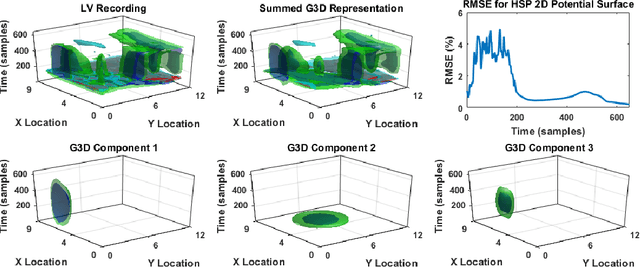
Objective: This paper proposes an neural network approach for predicting heart surface potentials (HSPs) from body surface potentials (BSPs), which reframes the traditional inverse problem of electrocardiography into a regression problem through the use of Gaussian 3D (G3D) basis function decomposition. Methods: HSPs were generated using G3D basis functions and passed through a boundary element forward model to obtain corresponding BSPs. The generated BSPs (input) and HSPs (output) were used to train a neural network, which was then used to predict a variety of synthesized and decomposed real-world HSPs. Results: Fitted G3D basis function parameters can accurately reconstruct the real-world left ventricular paced recording with percent root mean squared error (RMSE) of $1.34 \pm 1.30$%. The basis data trained neural network was able to predict G3D basis function synthesized data with RMSE of $8.46 \pm 1.55$%, and G3D representation of real-world data with RMSE of $18.5 \pm 5.25$%. Activation map produced from the predicted time series had a RMSE of 17.0% and mean absolute difference of $10.3 \pm 10.8$ms when compared to that produced from the actual left ventricular paced recording. Conclusion: A Gaussian basis function based data driven model for re-framing the inverse problem of electrocardiography as a regression problem is successful and produces promising time series and activation map predictions of real-world recordings even when only trained using Guassian data. Significance: The HSPs predicted by the neural network can be used to create activation maps to identify cardiac dysfunctions during clinical assessment.

A machine learning approach to reconstruction of heart surface potentials from body surface potentials

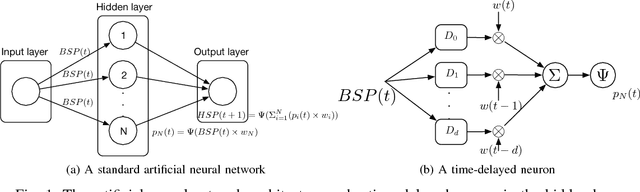
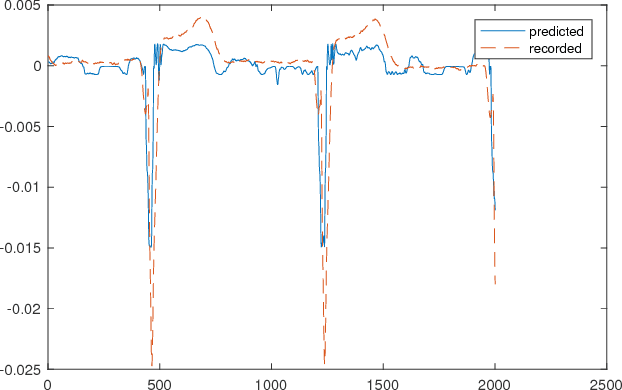
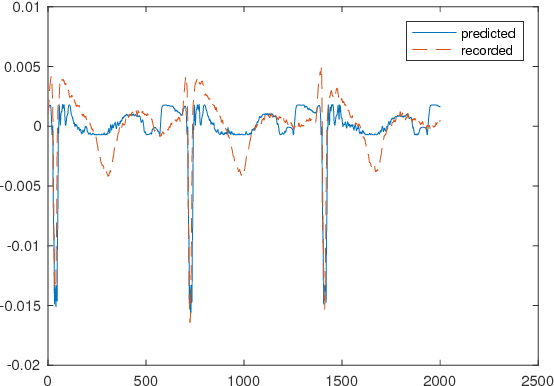
Invasive cardiac catheterisation is a common procedure that is carried out before surgical intervention. Yet, invasive cardiac diagnostics are full of risks, especially for young children. Decades of research has been conducted on the so called inverse problem of electrocardiography, which can be used to reconstruct Heart Surface Potentials (HSPs) from Body Surface Potentials (BSPs), for non-invasive diagnostics. State of the art solutions to the inverse problem are unsatisfactory, since the inverse problem is known to be ill-posed. In this paper we propose a novel approach to reconstructing HSPs from BSPs using a Time-Delay Artificial Neural Network (TDANN). We first design the TDANN architecture, and then develop an iterative search space algorithm to find the parameters of the TDANN, which results in the best overall HSP prediction. We use real-world recorded BSPs and HSPs from individuals suffering from serious cardiac conditions to validate our TDANN. The results are encouraging, in that coefficients obtained by correlating the predicted HSP with the recorded patient' HSP approach ideal values.