Use of Prior Knowledge to Discover Causal Additive Models with Unobserved Variables and its Application to Time Series Data

This paper proposes two methods for causal additive models with unobserved variables (CAM-UV). CAM-UV assumes that the causal functions take the form of generalized additive models and that latent confounders are present. First, we propose a method that leverages prior knowledge for efficient causal discovery. Then, we propose an extension of this method for inferring causality in time series data. The original CAM-UV algorithm differs from other existing causal function models in that it does not seek the causal order between observed variables, but rather aims to identify the causes for each observed variable. Therefore, the first proposed method in this paper utilizes prior knowledge, such as understanding that certain variables cannot be causes of specific others. Moreover, by incorporating the prior knowledge that causes precedes their effects in time, we extend the first algorithm to the second method for causal discovery in time series data. We validate the first proposed method by using simulated data to demonstrate that the accuracy of causal discovery increases as more prior knowledge is accumulated. Additionally, we test the second proposed method by comparing it with existing time series causal discovery methods, using both simulated data and real-world data.

Discovery of Causal Additive Models in the Presence of Unobserved Variables

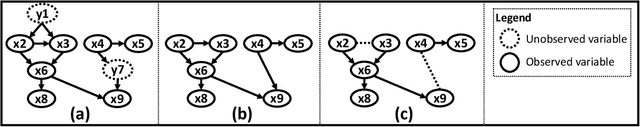
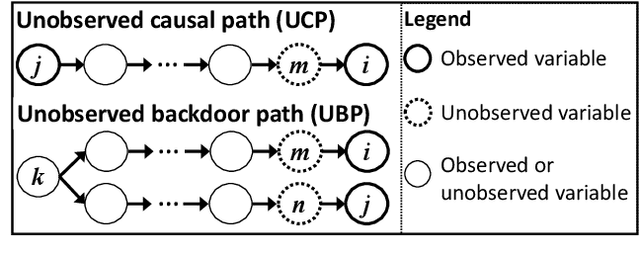
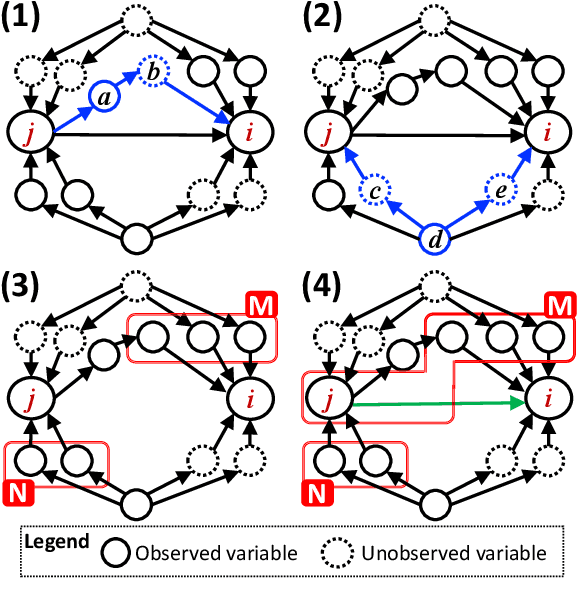
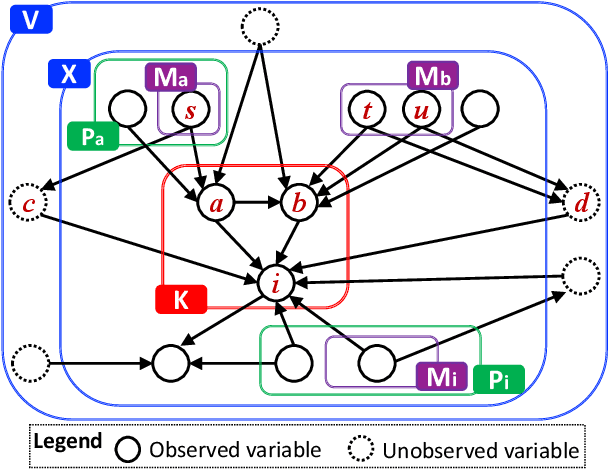
Causal discovery from data affected by unobserved variables is an important but difficult problem to solve. The effects that unobserved variables have on the relationships between observed variables are more complex in nonlinear cases than in linear cases. In this study, we focus on causal additive models in the presence of unobserved variables. Causal additive models exhibit structural equations that are additive in the variables and error terms. We take into account the presence of not only unobserved common causes but also unobserved intermediate variables. Our theoretical results show that, when the causal relationships are nonlinear and there are unobserved variables, it is not possible to identify all the causal relationships between observed variables through regression and independence tests. However, our theoretical results also show that it is possible to avoid incorrect inferences. We propose a method to identify all the causal relationships that are theoretically possible to identify without being biased by unobserved variables. The empirical results using artificial data and simulated functional magnetic resonance imaging (fMRI) data show that our method effectively infers causal structures in the presence of unobserved variables.

Causal discovery of linear non-Gaussian acyclic models in the presence of latent confounders

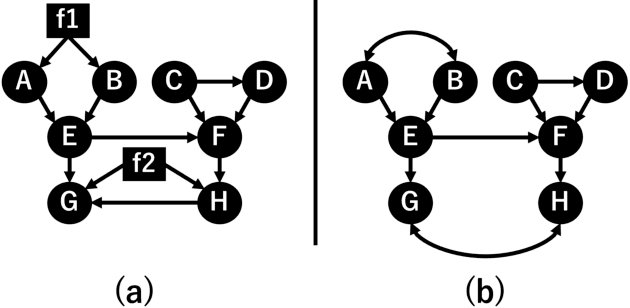
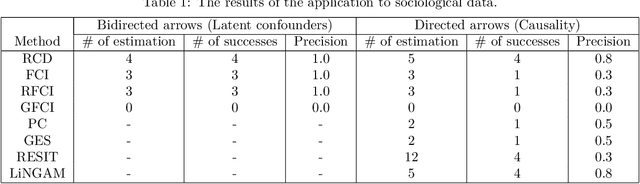
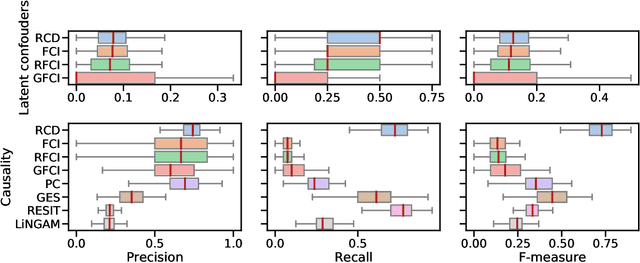
Causal discovery from data affected by latent confounders is an important and difficult challenge. Causal functional model-based approaches have not been used to present variables whose relationships are affected by latent confounders, while some constraint-based methods can present them. This paper proposes a causal functional model-based method called repetitive causal discovery (RCD) to discover the causal structure of observed variables affected by latent confounders. RCD repeats inferring the causal directions between a small number of observed variables and determines whether the relationships are affected by latent confounders. RCD finally produces a causal graph where a bi-directed arrow indicates the pair of variables that have the same latent confounders, and a directed arrow indicates the causal direction of a pair of variables that are not affected by the same latent confounder. The results of experimental validation using simulated data and real-world data confirmed that RCD is effective in identifying latent confounders and causal directions between observed variables.