Exact multiplicative updates for convolutional $β$-NMF in 2D

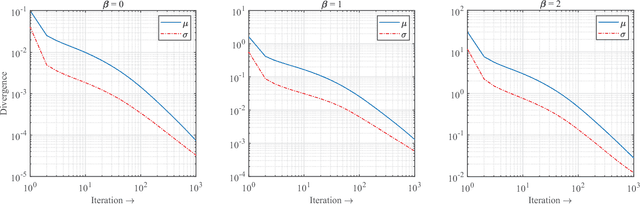
In this paper, we extend the $\beta$-CNMF to two dimensions and derive exact multiplicative updates for its factors. The new updates generalize and correct the nonnegative matrix factor deconvolution previously proposed by Schmidt and M{\o}rup. We show by simulation that the updates lead to a monotonically decreasing $\beta$-divergence in terms of the mean and the standard deviation and that the corresponding convergence curves are consistent across the most common values for $\beta$.

Multiplicative Updates for Convolutional NMF Under $β$-Divergence

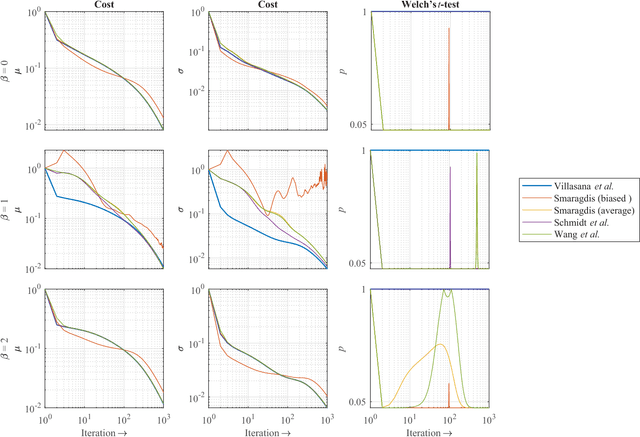
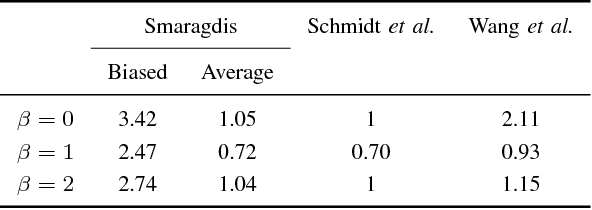
In this letter, we generalize the convolutional NMF by taking the $\beta$-divergence as the contrast function and present the correct multiplicative updates for its factors in closed form. The new updates unify the $\beta$-NMF and the convolutional NMF. We state why almost all of the existing updates are inexact and approximative w.r.t. the convolutional data model. We show that our updates are stable and that their convergence performance is consistent across the most common values of $\beta$.