Machine-Learned HASDM Model with Uncertainty Quantification

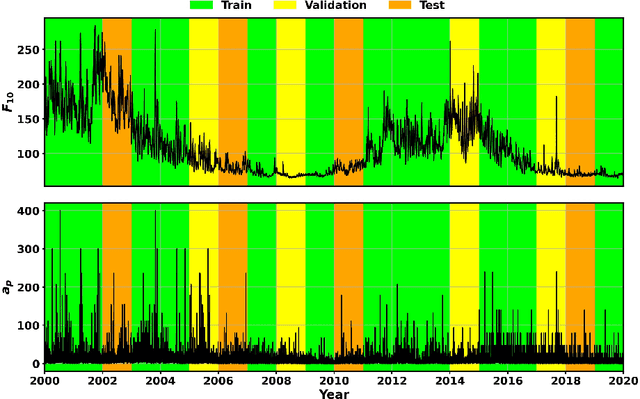
The first thermospheric neutral mass density model with robust and reliable uncertainty estimates is developed based on the SET HASDM density database. This database, created by Space Environment Technologies (SET), contains 20 years of outputs from the U.S. Space Force's High Accuracy Satellite Drag Model (HASDM), which represents the state-of-the-art for density and drag modeling. We utilize principal component analysis (PCA) for dimensionality reduction, creating the coefficients upon which nonlinear machine-learned (ML) regression models are trained. These models use three unique loss functions: mean square error (MSE), negative logarithm of predictive density (NLPD), and continuous ranked probability score (CRPS). Three input sets are also tested, showing improved performance when introducing time histories for geomagnetic indices. These models leverage Monte Carlo (MC) dropout to provide uncertainty estimates, and the use of the NLPD loss function results in well-calibrated uncertainty estimates without sacrificing model accuracy (<10% mean absolute error). By comparing the best HASDM-ML model to the HASDM database along satellite orbits, we found that the model provides robust and reliable uncertainties in the density space over all space weather conditions. A storm-time comparison shows that HASDM-ML also supplies meaningful uncertainty measurements during extreme events.

A landmark-based algorithm for automatic pattern recognition and abnormality detection

We study a class of mathematical and statistical algorithms with the aim of establishing a computer-based framework for fast and reliable automatic abnormality detection on landmark represented image templates. Under this framework, we apply a landmark-based algorithm for finding a group average as an estimator that is said to best represent the common features of the group in study. This algorithm extracts information of momentum at each landmark through the process of template matching. If ever converges, the proposed algorithm produces a local coordinate system for each member of the observing group, in terms of the residual momentum. We use a Bayesian approach on the collected residual momentum representations for making inference. For illustration, we apply this framework to a small database of brain images for detecting structure abnormality. The brain structure changes identified by our framework are highly consistent with studies in the literature.