Sébastien Cyr
Université de Montréal
Automating the Generation of High School Geometry Proofs using Prolog in an Educational Context
Feb 28, 2020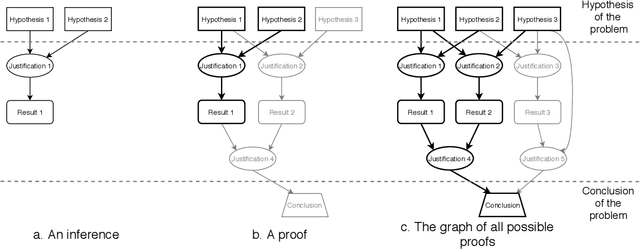
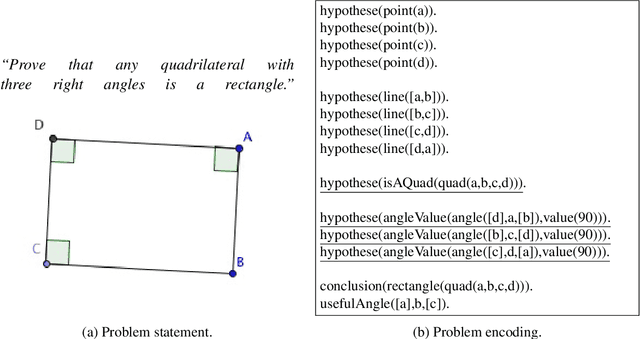

Abstract:When working on intelligent tutor systems designed for mathematics education and its specificities, an interesting objective is to provide relevant help to the students by anticipating their next steps. This can only be done by knowing, beforehand, the possible ways to solve a problem. Hence the need for an automated theorem prover that provide proofs as they would be written by a student. To achieve this objective, logic programming is a natural tool due to the similarity of its reasoning with a mathematical proof by inference. In this paper, we present the core ideas we used to implement such a prover, from its encoding in Prolog to the generation of the complete set of proofs. However, when dealing with educational aspects, there are many challenges to overcome. We also present the main issues we encountered, as well as the chosen solutions.
* In Proceedings ThEdu'19, arXiv:2002.11895
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge