Get our free extension to see links to code for papers anywhere online!Free add-on: code for papers everywhere!Free add-on: See code for papers anywhere!
Ryan Elmore
Keeping greed good: sparse regression under design uncertainty with application to biomass characterization
Jul 08, 2012Figures and Tables: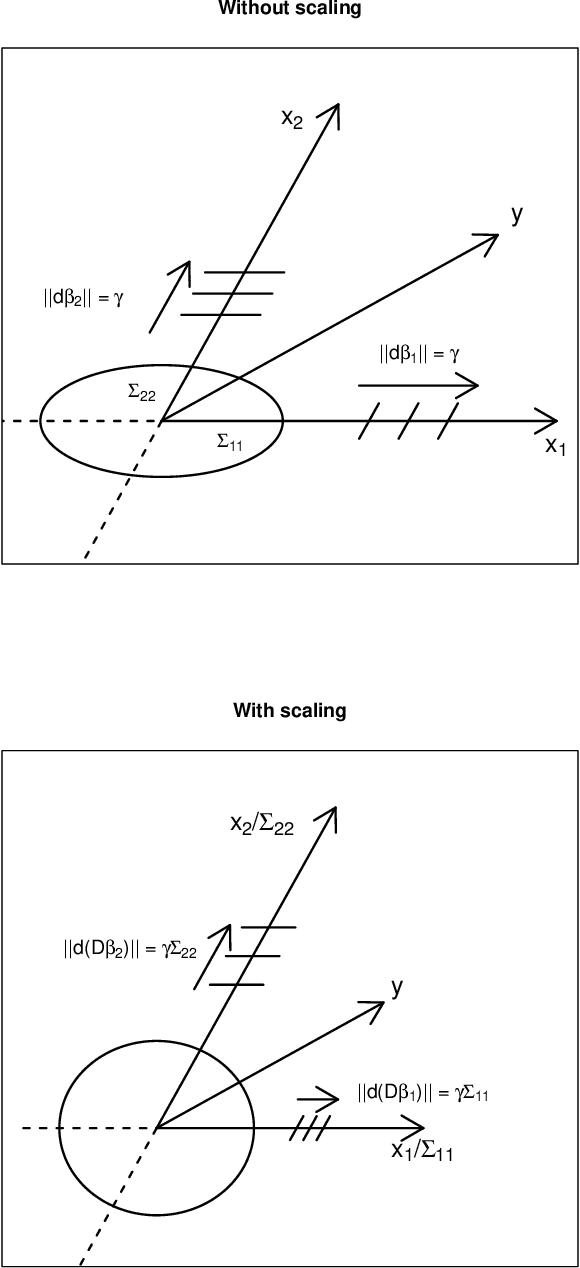

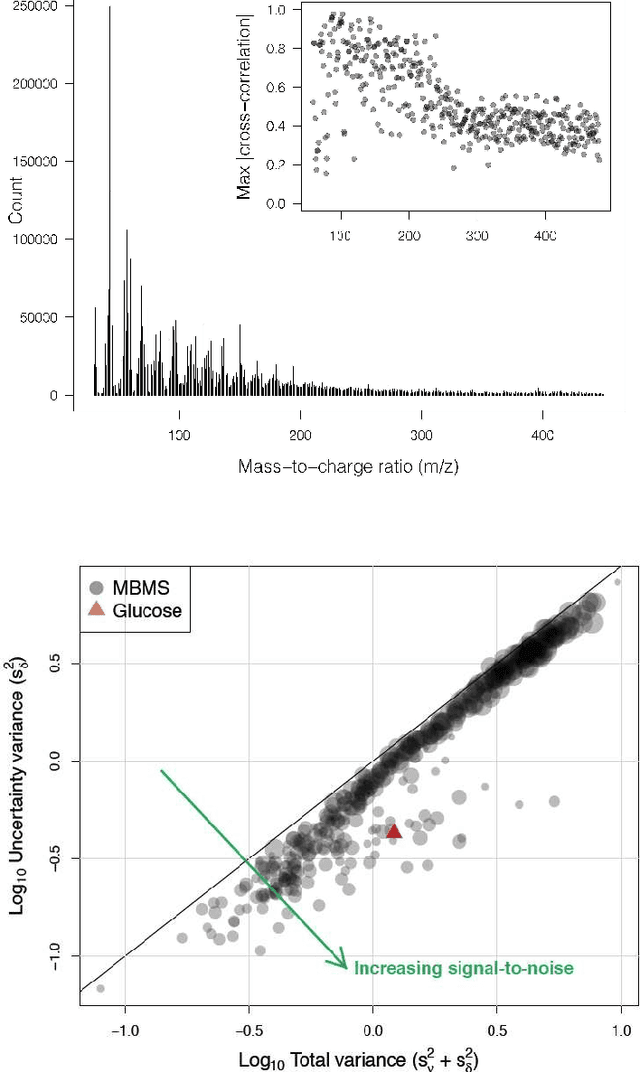
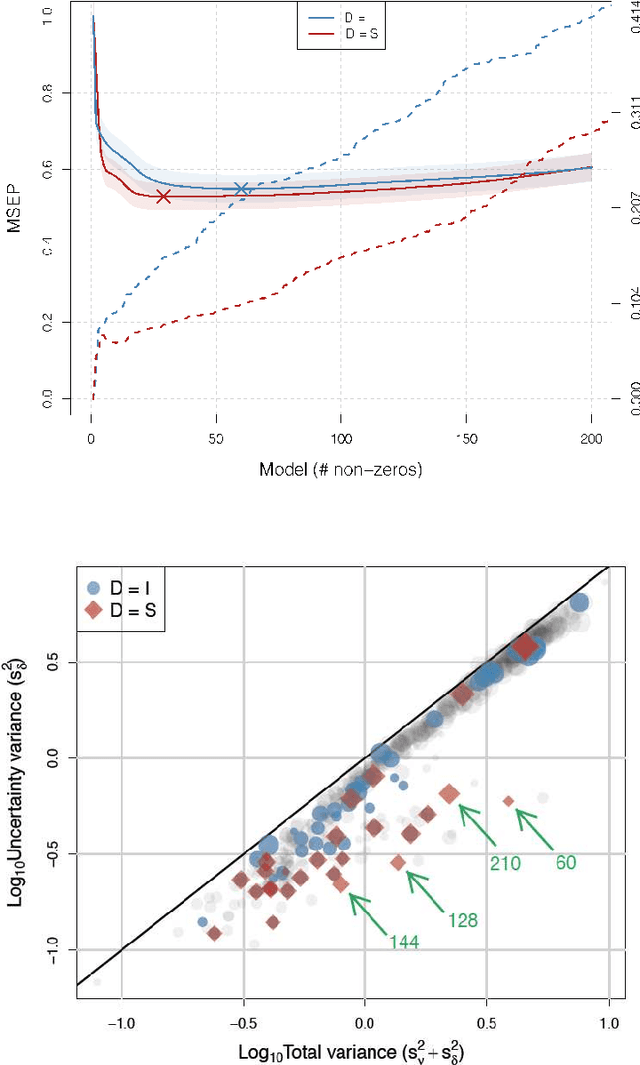




Abstract:In this paper, we consider the classic measurement error regression scenario in which our independent, or design, variables are observed with several sources of additive noise. We will show that our motivating example's replicated measurements on both the design and dependent variables may be leveraged to enhance a sparse regression algorithm. Specifically, we estimate the variance and use it to scale our design variables. We demonstrate the efficacy of scaling from several points of view and validate it empirically with a biomass characterization data set using two of the most widely used sparse algorithms: least angle regression (LARS) and the Dantzig selector (DS).
* 22 pages, 5 figures
Via
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge