Distribution of Classification Margins: Are All Data Equal?

Recent theoretical results show that gradient descent on deep neural networks under exponential loss functions locally maximizes classification margin, which is equivalent to minimizing the norm of the weight matrices under margin constraints. This property of the solution however does not fully characterize the generalization performance. We motivate theoretically and show empirically that the area under the curve of the margin distribution on the training set is in fact a good measure of generalization. We then show that, after data separation is achieved, it is possible to dynamically reduce the training set by more than 99% without significant loss of performance. Interestingly, the resulting subset of "high capacity" features is not consistent across different training runs, which is consistent with the theoretical claim that all training points should converge to the same asymptotic margin under SGD and in the presence of both batch normalization and weight decay.

Biologically Inspired Mechanisms for Adversarial Robustness

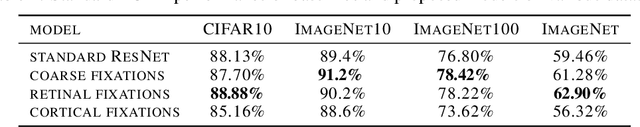
A convolutional neural network strongly robust to adversarial perturbations at reasonable computational and performance cost has not yet been demonstrated. The primate visual ventral stream seems to be robust to small perturbations in visual stimuli but the underlying mechanisms that give rise to this robust perception are not understood. In this work, we investigate the role of two biologically plausible mechanisms in adversarial robustness. We demonstrate that the non-uniform sampling performed by the primate retina and the presence of multiple receptive fields with a range of receptive field sizes at each eccentricity improve the robustness of neural networks to small adversarial perturbations. We verify that these two mechanisms do not suffer from gradient obfuscation and study their contribution to adversarial robustness through ablation studies.