Miklós Telek
Optimizing Pilot Spacing in MU-MIMO Systems Operating Over Aging Channels
Apr 01, 2022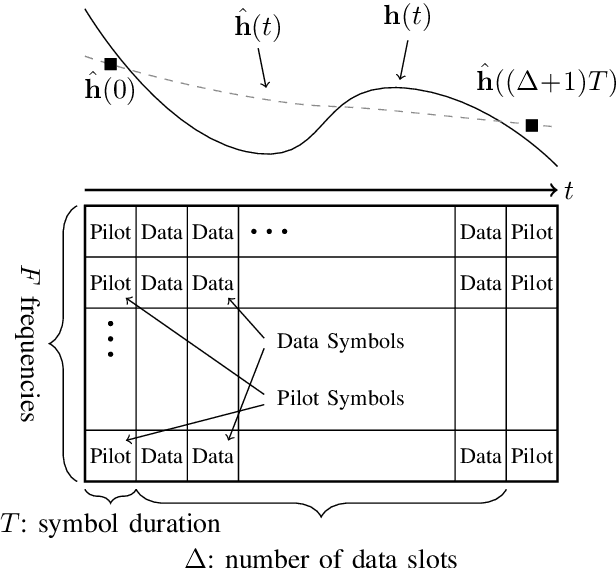
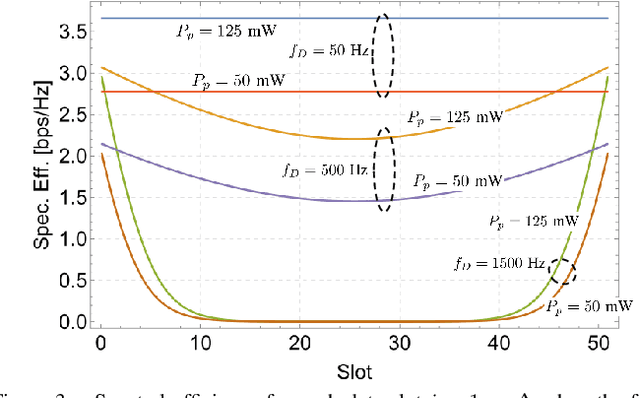
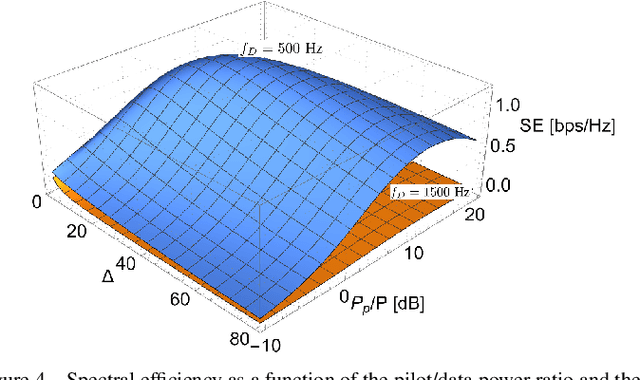
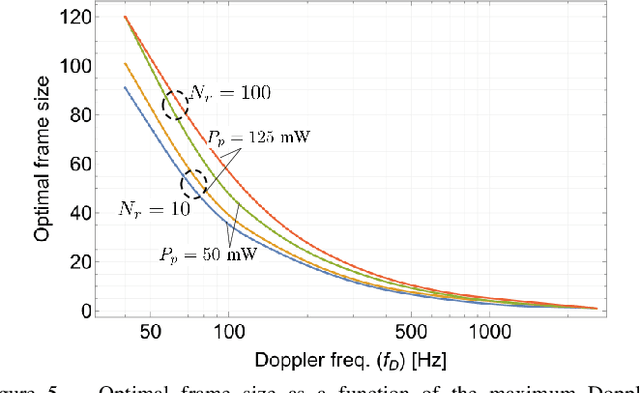
Abstract:In the uplink of multiuser multiple input multiple output (MU-MIMO) systems operating over aging channels, pilot spacing is crucial for acquiring channel state information and achieving high signal-to-interference-plus-noise ratio (SINR). Somewhat surprisingly, very few works examine the impact of pilot spacing on the correlation structure of subsequent channel estimates and the resulting quality of channel state information considering channel aging. In this paper, we consider a fast-fading environment characterized by its exponentially decaying autocorrelation function, and model pilot spacing as a sampling problem to capture the inherent trade-off between the quality of channel state information and the number of symbols available for information carrying data symbols. We first establish a quasi-closed form for the achievable asymptotic deterministic equivalent SINR when the channel estimation algorithm utilizes multiple pilot signals. Next, we establish upper bounds on the achievable SINR and spectral efficiency, as a function of pilot spacing, which helps to find the optimum pilot spacing within a limited search space. Our key insight is that to maximize the achievable SINR and the spectral efficiency of MU-MIMO systems, proper pilot spacing must be applied to control the impact of the aging channel and to tune the trade-off between pilot and data symbols.
On the Achievable SINR in MU-MIMO Systems Operating in Time-Varying Rayleigh Fading
Mar 24, 2022
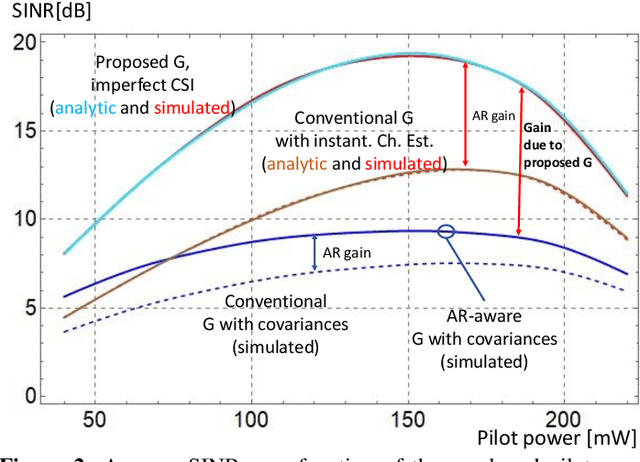
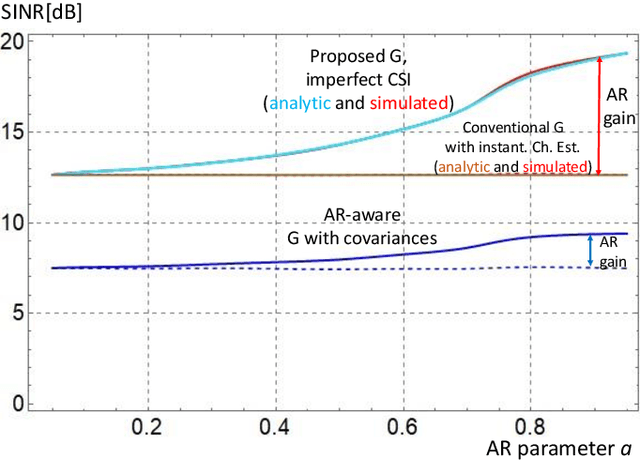

Abstract:Minimizing the symbol error in the uplink of multi-user multiple input multiple output systems is important, because the symbol error affects the achieved signal-to-interference-plus-noise ratio (SINR) and thereby the spectral efficiency of the system. Despite the vast literature available on minimum mean squared error (MMSE) receivers, previously proposed receivers for block fading channels do not minimize the symbol error in time-varying Rayleigh fading channels. Specifically, we show that the true MMSE receiver structure does not only depend on the statistics of the CSI error, but also on the autocorrelation coefficient of the time-variant channel. It turns out that calculating the average SINR when using the proposed receiver is highly non-trivial. In this paper, we employ a random matrix theoretical approach, which allows us to derive a quasi-closed form for the average SINR, which allows to obtain analytical exact results that give valuable insights into how the SINR depends on the number of antennas, employed pilot and data power and the covariance of the time-varying channel. We benchmark the performance of the proposed receiver against recently proposed receivers and find that the proposed MMSE receiver achieves higher SINR than the previously proposed ones, and this benefit increases with increasing autoregressive coefficient.
* 15 pages, 9 figures, 4 tables
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge