Using large language models for (de-)formalization and natural argumentation exercises for beginner's students

We describe two systems that use text-davinci-003, a large language model, for the automatized correction of (i) exercises in translating back and forth between natural language and the languages of propositional logic and first-order predicate logic and (ii) exercises in writing simple arguments in natural language in non-mathematical scenarios.

Improving the Diproche CNL through autoformalization via GPT-3

The Diproche system is an automated proof checker for texts written in a controlled fragment of German, designed for didactical applications in classes introducing students to proofs for the first time. The first version of the system used a controlled natural language for which a Prolog formalization routine was written. In this paper, we explore the possibility of prompting large language models for autoformalization in the context of Diproche, with encouraging first results.

Natural Language Proof Checking in Introduction to Proof Classes -- First Experiences with Diproche

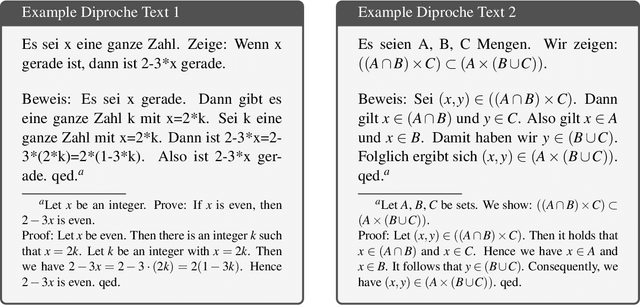
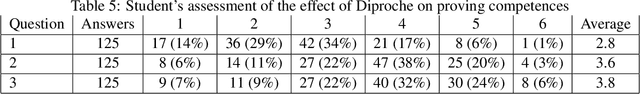
We present and analyze the employment of the Diproche system, a natural language proof checker, within a one-semester mathematics beginners lecture with 228 participants. The system is used to check the students' solution attempts to proving exercises in Boolean set theory and elementary number theory and to give them immediate feedback. The benefits of the employment of the system are assessed via a questionnaire at the end of the semester and via analyzing the solution attempts of a subgroup of the students. Based on our results we develop approaches for future improvements.

Automatized Evaluation of Formalization Exercises in Mathematics

We describe two systems for supporting beginner students in acquiring basic skills in expressing statements in the formalism of first-order predicate logic; the first, called "math dictations", presents users with the task of formalizing a given natural-language sentence, while the second, called "Game of Def", challenges users to give a formal description of a set of a geometric pattern displayed to them. In both cases, an automatic checking takes place.

Using Automated Theorem Provers for Mistake Diagnosis in the Didactics of Mathematics

The Diproche system, an automated proof checker for natural language proofs specifically adapted to the context of exercises for beginner's students similar to the Naproche system by Koepke, Schr\"oder, Cramer and others, uses a modification of an automated theorem prover which uses common formal fallacies intead of sound deduction rules for mistake diagnosis. We briefly describe the concept of such an `Anti-ATP' and explain the basic techniques used in its implementation.