The Block Point Process Model for Continuous-Time Event-Based Dynamic Networks

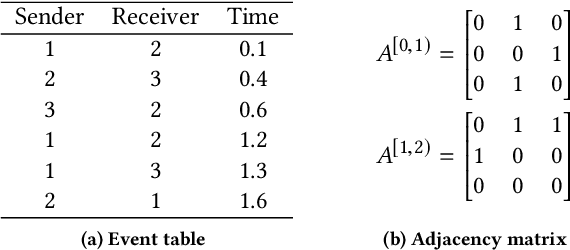
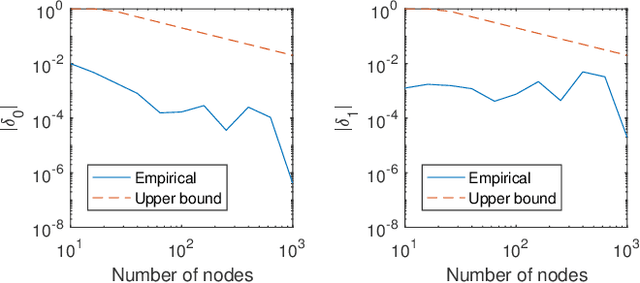
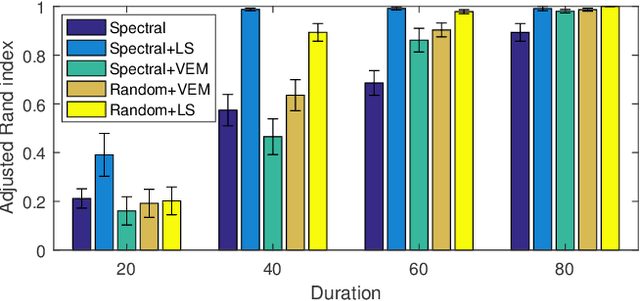
Many application settings involve the analysis of timestamped relations or events between a set of entities, e.g. messages between users of an on-line social network. Static and discrete-time network models are typically used as analysis tools in these settings; however, they discard a significant amount of information by aggregating events over time to form network snapshots. In this paper, we introduce a block point process model (BPPM) for dynamic networks evolving in continuous time in the form of events at irregular time intervals. The BPPM is inspired by the well-known stochastic block model (SBM) for static networks and is a simpler version of the recently-proposed Hawkes infinite relational model (IRM). We show that networks generated by the BPPM follow an SBM in the limit of a growing number of nodes and leverage this property to develop an efficient inference procedure for the BPPM. We fit the BPPM to several real network data sets, including a Facebook network with over 3, 500 nodes and 130, 000 events, several orders of magnitude larger than the Hawkes IRM and other existing point process network models.

Unsupervised Motion Artifact Detection in Wrist-Measured Electrodermal Activity Data

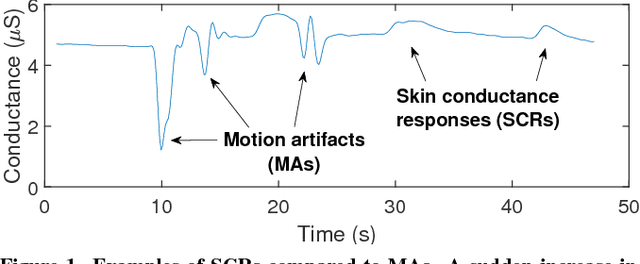
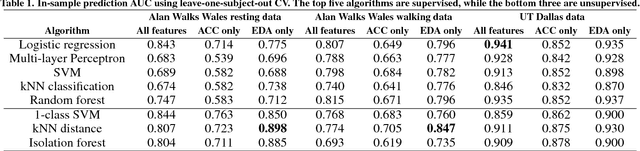
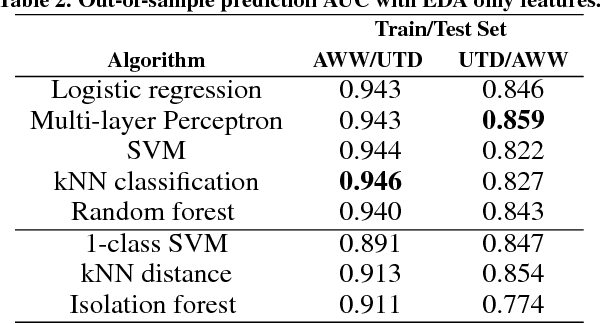
One of the main benefits of a wrist-worn computer is its ability to collect a variety of physiological data in a minimally intrusive manner. Among these data, electrodermal activity (EDA) is readily collected and provides a window into a person's emotional and sympathetic responses. EDA data collected using a wearable wristband are easily influenced by motion artifacts (MAs) that may significantly distort the data and degrade the quality of analyses performed on the data if not identified and removed. Prior work has demonstrated that MAs can be successfully detected using supervised machine learning algorithms on a small data set collected in a lab setting. In this paper, we demonstrate that unsupervised learning algorithms perform competitively with supervised algorithms for detecting MAs on EDA data collected in both a lab-based setting and a real-world setting comprising about 23 hours of data. We also find, somewhat surprisingly, that incorporating accelerometer data as well as EDA improves detection accuracy only slightly for supervised algorithms and significantly degrades the accuracy of unsupervised algorithms.