Attribute Exploration with Multiple Contradicting Partial Experts

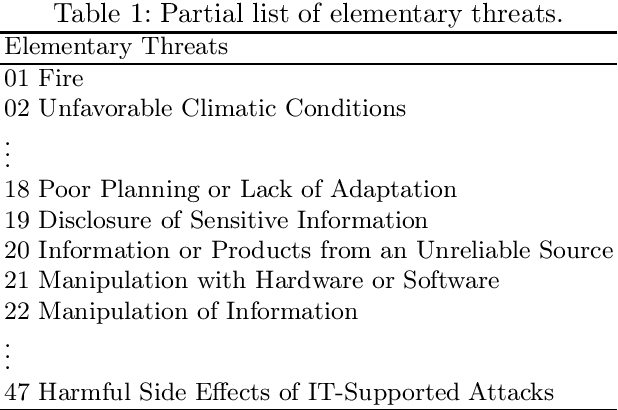
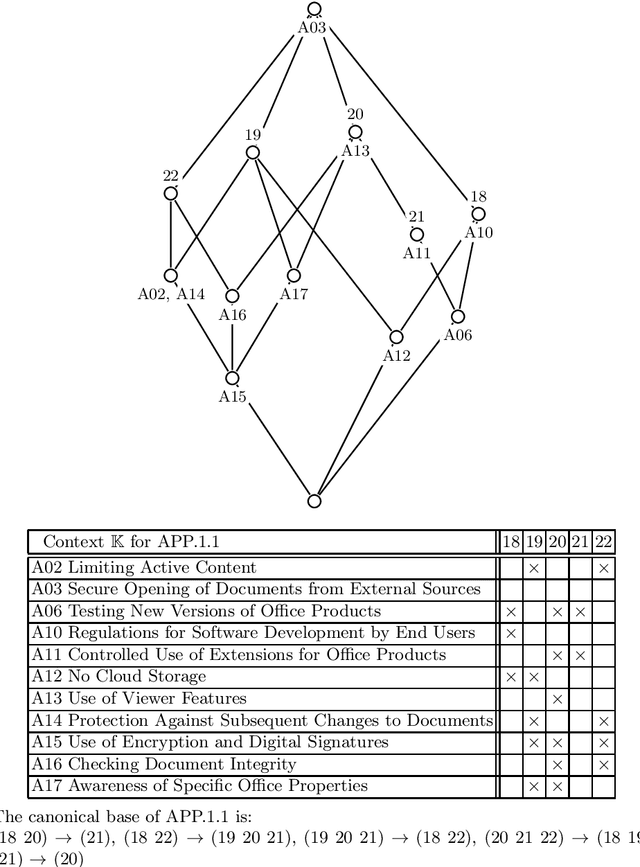
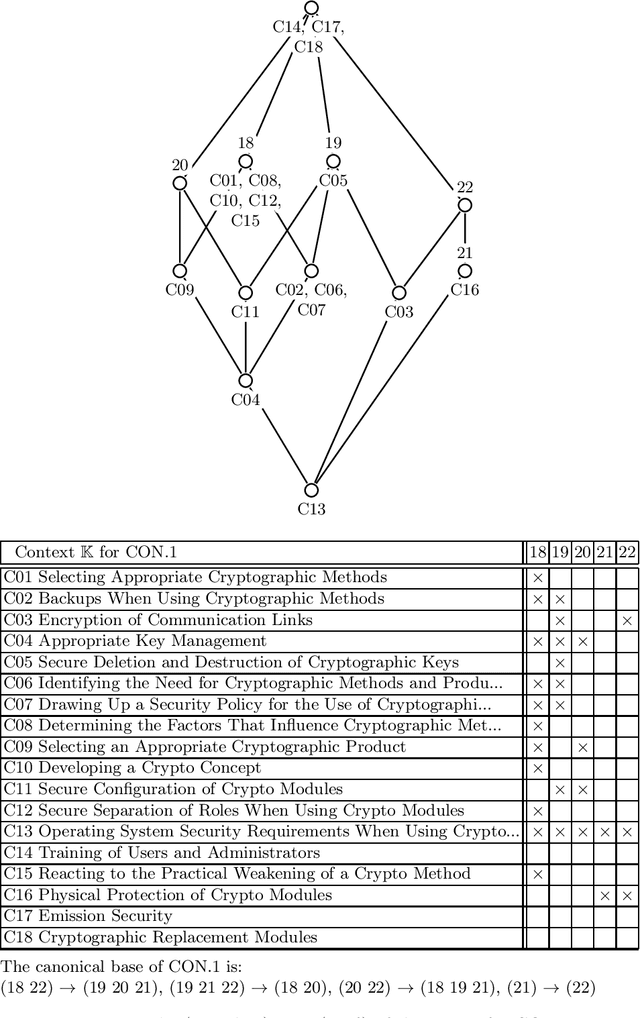
Attribute exploration is a method from Formal Concept Analysis (FCA) that helps a domain expert discover structural dependencies in knowledge domains which can be represented as formal contexts (cross tables of objects and attributes). In this paper we present an extension of attribute exploration that allows for a group of domain experts and explores their shared views. Each expert has their own view of the domain and the views of multiple experts may contain contradicting information.

Triadic Exploration and Exploration with Multiple Experts

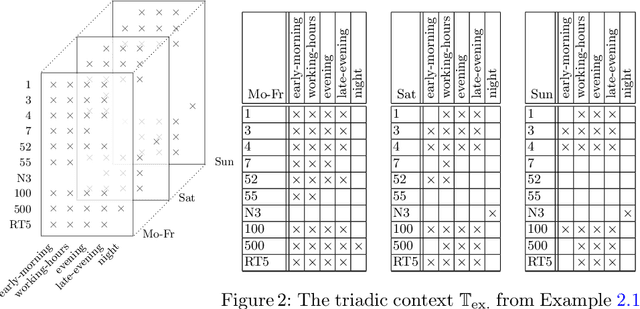
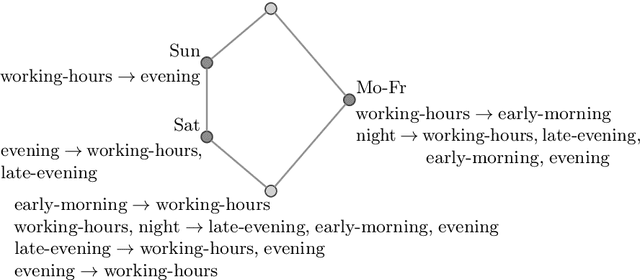
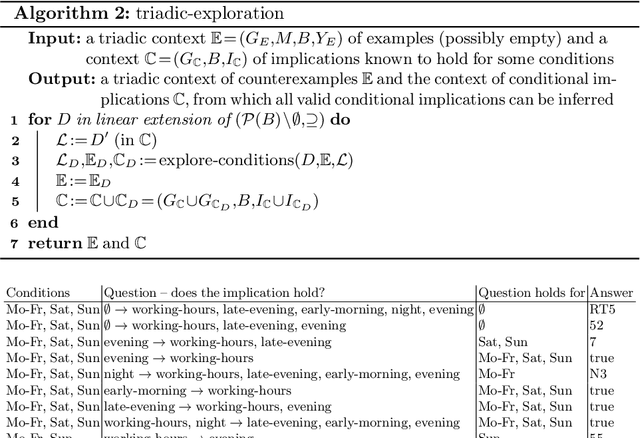
Formal Concept Analysis (FCA) provides a method called attribute exploration which helps a domain expert discover structural dependencies in knowledge domains that can be represented by a formal context (a cross table of objects and attributes). Triadic Concept Analysis is an extension of FCA that incorporates the notion of conditions. Many extensions and variants of attribute exploration have been studied but only few attempts at incorporating multiple experts have been made. In this paper we present triadic exploration based on Triadic Concept Analysis to explore conditional attribute implications in a triadic domain. We then adapt this approach to formulate attribute exploration with multiple experts that have different views on a domain.

Interactive Collaborative Exploration using Incomplete Contexts

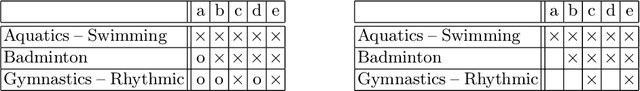
A common representation of information about relations of objects and attributes in knowledge domains are data-tables. The structure of such information can be analysed using Formal Concept Analysis (FCA). Attribute exploration is a knowledge acquisition method from FCA that reveals dependencies in a set of attributes with help of a domain expert. However, in general no single expert is capable (time- and knowledge-wise) of exploring knowledge domains alone. Therefore it is important to develop methods that allow multiple experts to explore domains together. To this end we build upon results on representation of incomplete knowledge [2, 8-10], adapt the corresponding version of attribute exploration to fit the setting of multiple experts and suggest formalizations for key components like expert knowledge, interaction and collaboration strategy. Furthermore we discuss ways of comparing collaboration strategies and suggest avenues for future research.

Formal Context Generation using Dirichlet Distributions

We suggest an improved way to randomly generate formal contexts based on Dirichlet distributions. For this purpose we investigate the predominant way to generate formal contexts, a coin-tossing model, recapitulate some of its shortcomings and examine its stochastic model. Building up on this we propose our Dirichlet model and develop an algorithm employing this idea. By comparing our generation model to a coin-tossing model we show that our approach is a significant improvement with respect to the variety of contexts generated. Finally, we outline a possible application in null model generation for formal contexts.