Reasoning by Cases in Structured Argumentation

We extend the $ASPIC^+$ framework for structured argumentation so as to allow applications of the reasoning by cases inference scheme for defeasible arguments. Given an argument with conclusion `$A$ or $B$', an argument based on $A$ with conclusion $C$, and an argument based on $B$ with conclusion $C$, we allow the construction of an argument with conclusion $C$. We show how our framework leads to different results than other approaches in non-monotonic logic for dealing with disjunctive information, such as disjunctive default theory or approaches based on the OR-rule (which allows to derive a defeasible rule `If ($A$ or $B$) then $C$', given two defeasible rules `If $A$ then $C$' and `If $B$ then $C$'). We raise new questions regarding the subtleties of reasoning defeasibly with disjunctive information, and show that its formalization is more intricate than one would presume.

A structured argumentation framework for detaching conditional obligations

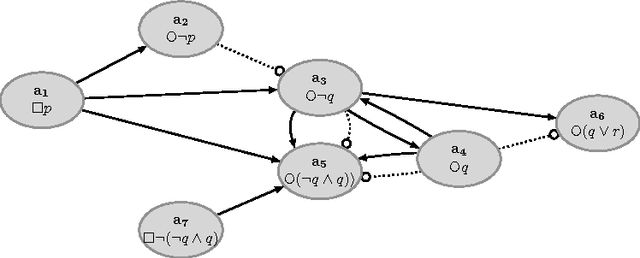
We present a general formal argumentation system for dealing with the detachment of conditional obligations. Given a set of facts, constraints, and conditional obligations, we answer the question whether an unconditional obligation is detachable by considering reasons for and against its detachment. For the evaluation of arguments in favor of detaching obligations we use a Dung-style argumentation-theoretical semantics. We illustrate the modularity of the general framework by considering some extensions, and we compare the framework to some related approaches from the literature.