Structure Learning of Markov Random Fields through Grow-Shrink Maximum Pseudolikelihood Estimation

Learning the structure of Markov random fields (MRFs) plays an important role in multivariate analysis. The importance has been increasing with the recent rise of statistical relational models since the MRF serves as a building block of these models such as Markov logic networks. There are two fundamental ways to learn structures of MRFs: methods based on parameter learning and those based on independence test. The former methods more or less assume certain forms of distribution, so they potentially perform poorly when the assumption is not satisfied. The latter can learn an MRF structure without a strong distributional assumption, but sometimes it is unclear what objective function is maximized/minimized in these methods. In this paper, we follow the latter, but we explicitly define the optimization problem of MRF structure learning as maximum pseudolikelihood estimation (MPLE) with respect to the edge set. As a result, the proposed solution successfully deals with the {\em symmetricity} in MRFs, whereas such symmetricity is not taken into account in most existing independence test techniques. The proposed method achieved higher accuracy than previous methods when there were asymmetric dependencies in our experiments.

Posterior Mean Super-resolution with a Causal Gaussian Markov Random Field Prior

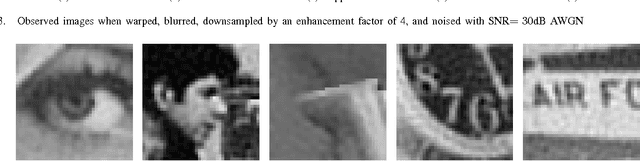
We propose a Bayesian image super-resolution (SR) method with a causal Gaussian Markov random field (MRF) prior. SR is a technique to estimate a spatially high-resolution image from given multiple low-resolution images. An MRF model with the line process supplies a preferable prior for natural images with edges. We improve the existing image transformation model, the compound MRF model, and its hyperparameter prior model. We also derive the optimal estimator -- not the joint maximum a posteriori (MAP) or marginalized maximum likelihood (ML), but the posterior mean (PM) -- from the objective function of the L2-norm (mean square error) -based peak signal-to-noise ratio (PSNR). Point estimates such as MAP and ML are generally not stable in ill-posed high-dimensional problems because of overfitting, while PM is a stable estimator because all the parameters in the model are evaluated as distributions. The estimator is numerically determined by using variational Bayes. Variational Bayes is a widely used method that approximately determines a complicated posterior distribution, but it is generally hard to use because it needs the conjugate prior. We solve this problem with simple Taylor approximations. Experimental results have shown that the proposed method is more accurate or comparable to existing methods.

Posterior Mean Super-Resolution with a Compound Gaussian Markov Random Field Prior

This manuscript proposes a posterior mean (PM) super-resolution (SR) method with a compound Gaussian Markov random field (MRF) prior. SR is a technique to estimate a spatially high-resolution image from observed multiple low-resolution images. A compound Gaussian MRF model provides a preferable prior for natural images that preserves edges. PM is the optimal estimator for the objective function of peak signal-to-noise ratio (PSNR). This estimator is numerically determined by using variational Bayes (VB). We then solve the conjugate prior problem on VB and the exponential-order calculation cost problem of a compound Gaussian MRF prior with simple Taylor approximations. In experiments, the proposed method roughly overcomes existing methods.