Jan van Eijck
Belief as Willingness to Bet
Dec 17, 2014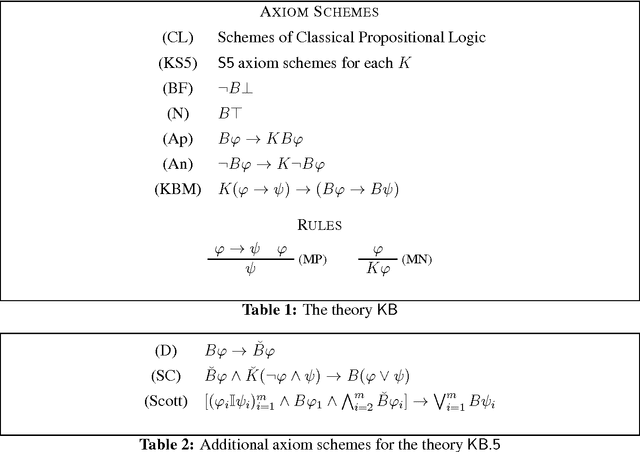
Abstract:We investigate modal logics of high probability having two unary modal operators: an operator $K$ expressing probabilistic certainty and an operator $B$ expressing probability exceeding a fixed rational threshold $c\geq\frac 12$. Identifying knowledge with the former and belief with the latter, we may think of $c$ as the agent's betting threshold, which leads to the motto "belief is willingness to bet." The logic $\mathsf{KB.5}$ for $c=\frac 12$ has an $\mathsf{S5}$ $K$ modality along with a sub-normal $B$ modality that extends the minimal modal logic $\mathsf{EMND45}$ by way of four schemes relating $K$ and $B$, one of which is a complex scheme arising out of a theorem due to Scott. Lenzen was the first to use Scott's theorem to show that a version of this logic is sound and complete for the probability interpretation. We reformulate Lenzen's results and present them here in a modern and accessible form. In addition, we introduce a new epistemic neighborhood semantics that will be more familiar to modern modal logicians. Using Scott's theorem, we provide the Lenzen-derivative properties that must be imposed on finite epistemic neighborhood models so as to guarantee the existence of a probability measure respecting the neighborhood function in the appropriate way for threshold $c=\frac 12$. This yields a link between probabilistic and modal neighborhood semantics that we hope will be of use in future work on modal logics of qualitative probability. We leave open the question of which properties must be imposed on finite epistemic neighborhood models so as to guarantee existence of an appropriate probability measure for thresholds $c\neq\frac 12$.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge