Operating data of a specific Aquatic Center as a Benchmark for dynamic model learning: search for a valid prediction model over an 8-hour horizon

This paper presents an identification repository based on data from a public swimming pool in operation. Such a system is both a complex process and easily understandable by all with regard to the issues. Ultimately, the aim is to reduce the energy bill while maintaining the level of quality of service. This objective is general in scope and not just limited to public swimming pools. It can be done efficiently through what is known as economic predictive control. This type of advanced control is based on a process model. It is the problem of this article and the benchmark considered to show that such a dynamic model can be obtained from operating data. For this, operational data is formatted and shared, and model quality indicators are proposed. On this basis, the first identification results illustrate the results obtained by a linear multivariable model on the one hand, and by a neural model on the other hand. They call for other proposals and results from control and data scientists for comparison.

Relational Constraints for Metric Learning on Relational Data

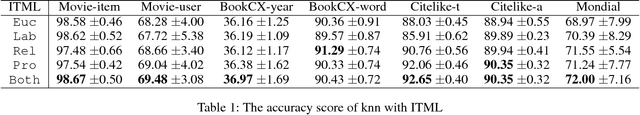
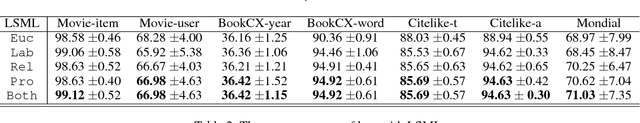
Most of metric learning approaches are dedicated to be applied on data described by feature vectors, with some notable exceptions such as times series, trees or graphs. The objective of this paper is to propose a metric learning algorithm that specifically considers relational data. The proposed approach can take benefit from both the topological structure of the data and supervised labels. For selecting relative constraints representing the relational information, we introduce a link-strength function that measures the strength of relationship links between entities by the side-information of their common parents. We show the performance of the proposed method with two different classical metric learning algorithms, which are ITML (Information Theoretic Metric Learning) and LSML (Least Squares Metric Learning), and test on several real-world datasets. Experimental results show that using relational information improves the quality of the learned metric.

Constraint Selection in Metric Learning

A number of machine learning algorithms are using a metric, or a distance, in order to compare individuals. The Euclidean distance is usually employed, but it may be more efficient to learn a parametric distance such as Mahalanobis metric. Learning such a metric is a hot topic since more than ten years now, and a number of methods have been proposed to efficiently learn it. However, the nature of the problem makes it quite difficult for large scale data, as well as data for which classes overlap. This paper presents a simple way of improving accuracy and scalability of any iterative metric learning algorithm, where constraints are obtained prior to the algorithm. The proposed approach relies on a loss-dependent weighted selection of constraints that are used for learning the metric. Using the corresponding dedicated loss function, the method clearly allows to obtain better results than state-of-the-art methods, both in terms of accuracy and time complexity. Some experimental results on real world, and potentially large, datasets are demonstrating the effectiveness of our proposition.