Erik Scharwächter
Statistical Evaluation of Anomaly Detectors for Sequences
Aug 13, 2020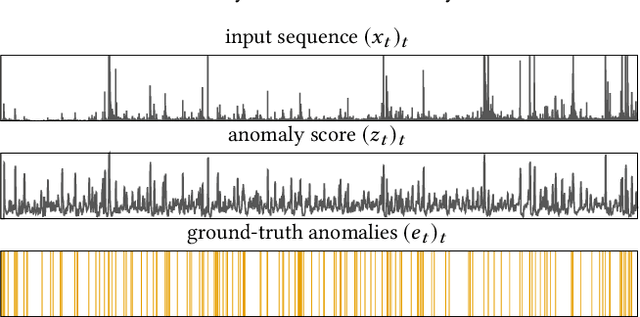
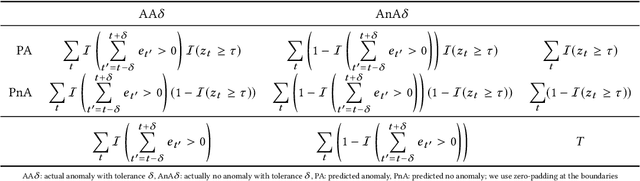
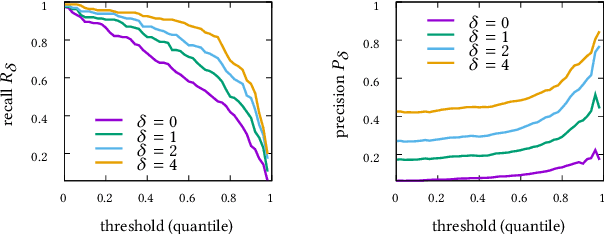

Abstract:Although precision and recall are standard performance measures for anomaly detection, their statistical properties in sequential detection settings are poorly understood. In this work, we formalize a notion of precision and recall with temporal tolerance for point-based anomaly detection in sequential data. These measures are based on time-tolerant confusion matrices that may be used to compute time-tolerant variants of many other standard measures. However, care has to be taken to preserve interpretability. We perform a statistical simulation study to demonstrate that precision and recall may overestimate the performance of a detector, when computed with temporal tolerance. To alleviate this problem, we show how to obtain null distributions for the two measures to assess the statistical significance of reported results.
Differentiable Segmentation of Sequences
Jun 23, 2020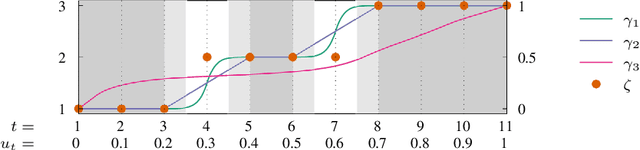
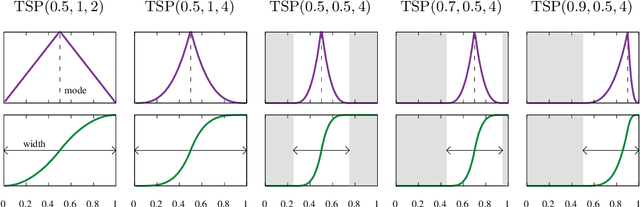
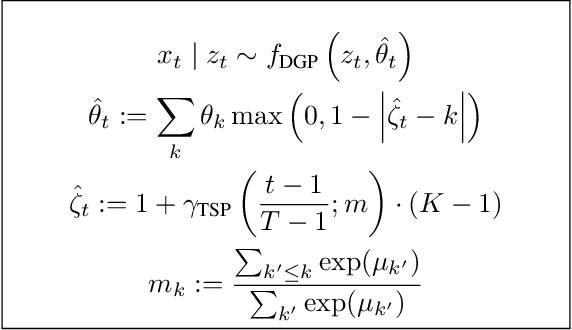
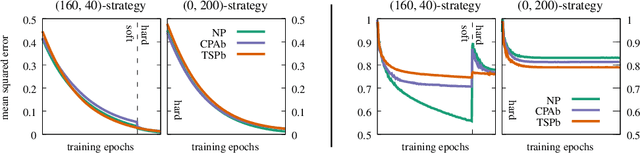
Abstract:Segmented models are widely used to describe non-stationary sequential data with discrete change points. Their estimation usually requires solving a mixed discrete-continuous optimization problem, where the segmentation is the discrete part and all other model parameters are continuous. A number of estimation algorithms have been developed that are highly specialized for their specific model assumptions. The dependence on non-standard algorithms makes it hard to integrate segmented models in state-of-the-art deep learning architectures that critically depend on gradient-based optimization techniques. In this work, we formulate a relaxed variant of segmented models that enables joint estimation of all model parameters, including the segmentation, with gradient descent. We build on recent advances in learning continuous warping functions and propose a novel family of warping functions based on the two-sided power (TSP) distribution. TSP-based warping functions are differentiable, have simple closed-form expressions, and can represent segmentation functions exactly. Our formulation includes the important class of segmented generalized linear models as a special case, which makes it highly versatile. We use our approach to model the spread of COVID-19 by segmented Poisson regression, perform logistic regression on Fashion-MNIST with artificial concept drift, and demonstrate its capacities for phoneme segmentation.
Does Terrorism Trigger Online Hate Speech? On the Association of Events and Time Series
Apr 30, 2020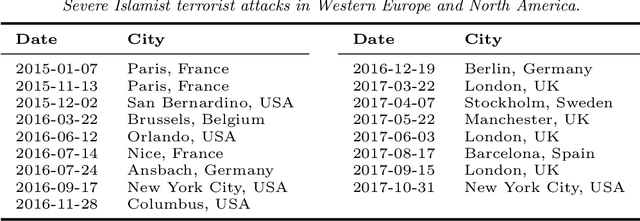
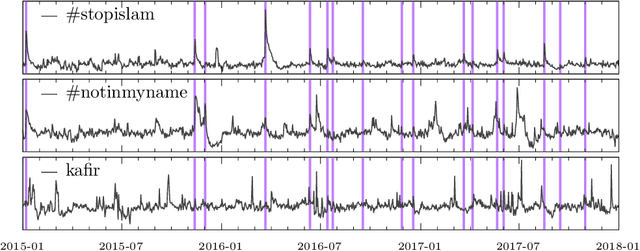
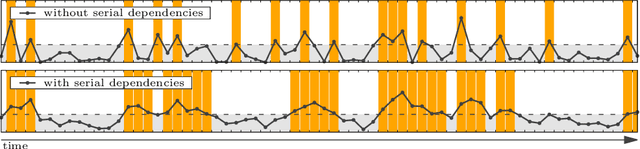
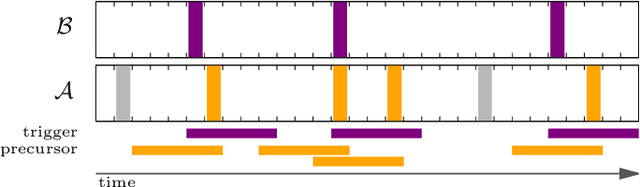
Abstract:Hate speech is ubiquitous on the Web. Recently, the offline causes that contribute to online hate speech have received increasing attention. A recurring question is whether the occurrence of extreme events offline systematically triggers bursts of hate speech online, indicated by peaks in the volume of hateful social media posts. Formally, this question translates into measuring the association between a sparse event series and a time series. We propose a novel statistical methodology to measure, test and visualize the systematic association between rare events and peaks in a time series. In contrast to previous methods for causal inference or independence tests on time series, our approach focuses only on the timing of events and peaks, and no other distributional characteristics. We follow the framework of event coincidence analysis (ECA) that was originally developed to correlate point processes. We formulate a discrete-time variant of ECA and derive all required distributions to enable analyses of peaks in time series, with a special focus on serial dependencies and peaks over multiple thresholds. The analysis gives rise to a novel visualization of the association via quantile-trigger rate plots. We demonstrate the utility of our approach by analyzing whether Islamist terrorist attacks in Western Europe and North America systematically trigger bursts of hate speech and counter-hate speech on Twitter.
Two-Sample Testing for Event Impacts in Time Series
Jan 31, 2020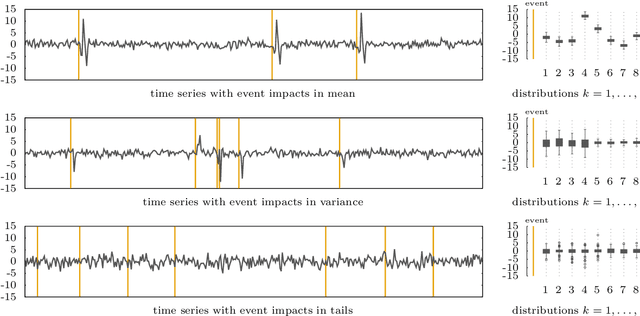
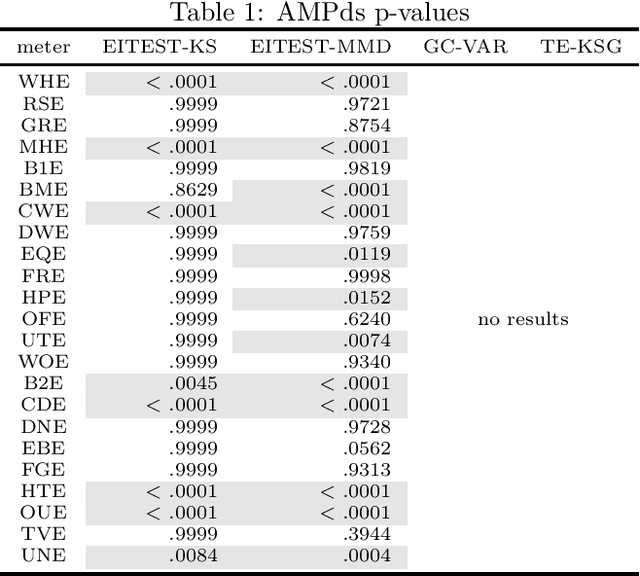
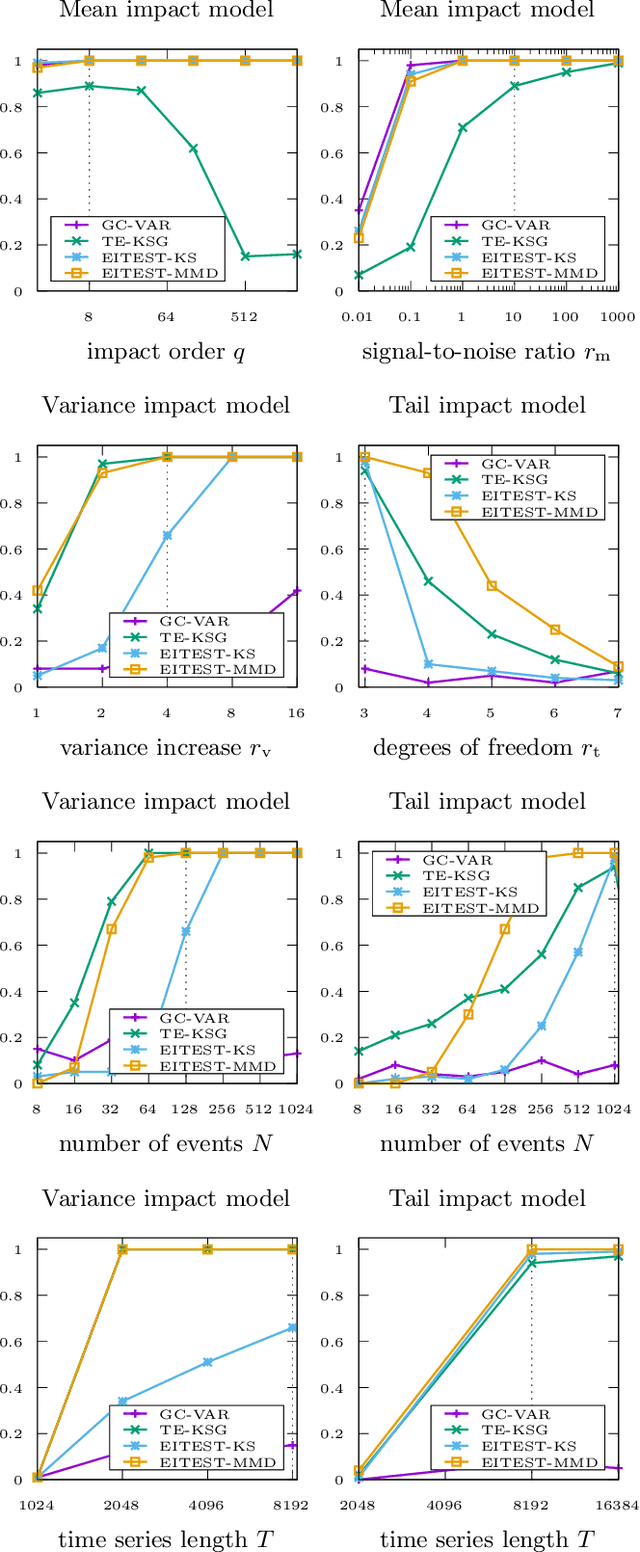
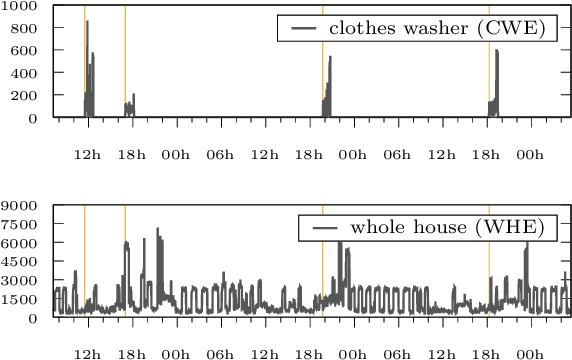
Abstract:In many application domains, time series are monitored to detect extreme events like technical faults, natural disasters, or disease outbreaks. Unfortunately, it is often non-trivial to select both a time series that is informative about events and a powerful detection algorithm: detection may fail because the detection algorithm is not suitable, or because there is no shared information between the time series and the events of interest. In this work, we thus propose a non-parametric statistical test for shared information between a time series and a series of observed events. Our test allows identifying time series that carry information on event occurrences without committing to a specific event detection methodology. In a nutshell, we test for divergences of the value distributions of the time series at increasing lags after event occurrences with a multiple two-sample testing approach. In contrast to related tests, our approach is applicable for time series over arbitrary domains, including multivariate numeric, strings or graphs. We perform a large-scale simulation study to show that it outperforms or is on par with related tests on our task for univariate time series. We also demonstrate the real-world applicability of our approach on datasets from social media and smart home environments.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge