Handling boundary constraints for numerical optimization by particle swarm flying in periodic search space

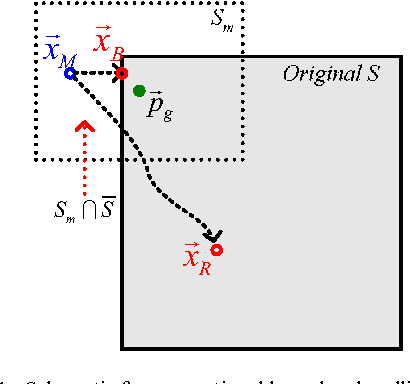
The periodic mode is analyzed together with two conventional boundary handling modes for particle swarm. By providing an infinite space that comprises periodic copies of original search space, it avoids possible disorganizing of particle swarm that is induced by the undesired mutations at the boundary. The results on benchmark functions show that particle swarm with periodic mode is capable of improving the search performance significantly, by compared with that of conventional modes and other algorithms.

Handling equality constraints by adaptive relaxing rule for swarm algorithms

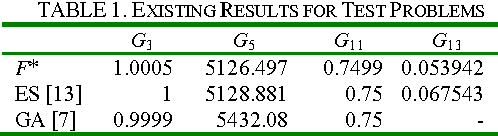
The adaptive constraints relaxing rule for swarm algorithms to handle with the problems with equality constraints is presented. The feasible space of such problems may be similiar to ridge function class, which is hard for applying swarm algorithms. To enter the solution space more easily, the relaxed quasi feasible space is introduced and shrinked adaptively. The experimental results on benchmark functions are compared with the performance of other algorithms, which show its efficiency.

Optimizing semiconductor devices by self-organizing particle swarm

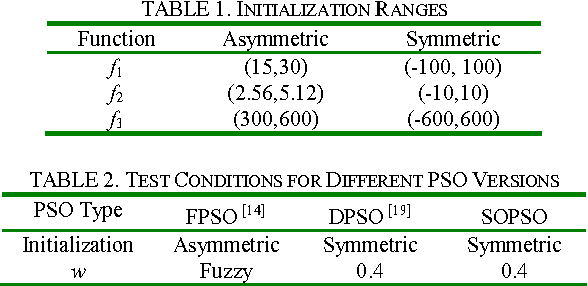
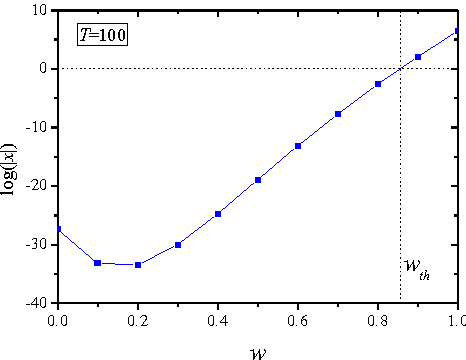
A self-organizing particle swarm is presented. It works in dissipative state by employing the small inertia weight, according to experimental analysis on a simplified model, which with fast convergence. Then by recognizing and replacing inactive particles according to the process deviation information of device parameters, the fluctuation is introduced so as to driving the irreversible evolution process with better fitness. The testing on benchmark functions and an application example for device optimization with designed fitness function indicates it improves the performance effectively.