David M Umulis
PDE-constrained shape registration to characterize biological growth and morphogenesis from imaging data
Apr 05, 2022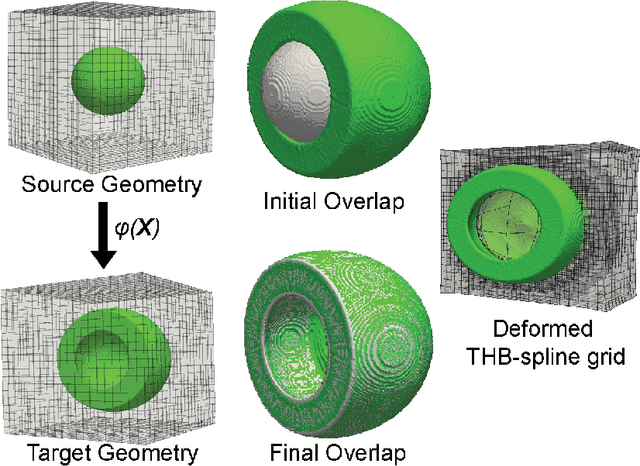
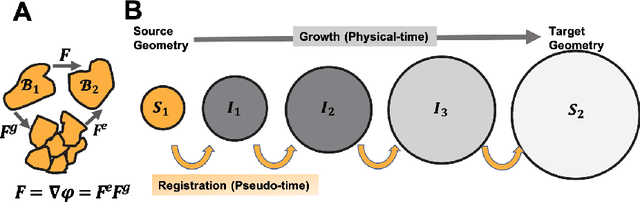
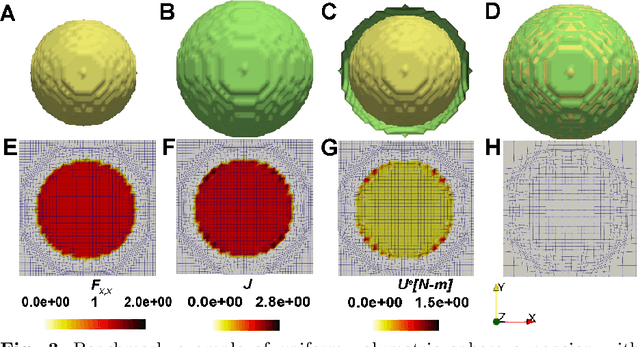

Abstract:We propose a PDE-constrained shape registration algorithm that captures the deformation and growth of biological tissue from imaging data. Shape registration is the process of evaluating optimum alignment between pairs of geometries through a spatial transformation function. We start from our previously reported work, which uses 3D tensor product B-spline basis functions to interpolate 3D space. Here, the movement of the B-spline control points, composed with an implicit function describing the shape of the tissue, yields the total deformation gradient field. The deformation gradient is then split into growth and elastic contributions. The growth tensor captures addition of mass, i.e. growth, and evolves according to a constitutive equation which is usually a function of the elastic deformation. Stress is generated in the material due to the elastic component of the deformation alone. The result of the registration is obtained by minimizing a total energy functional which includes: a distance measure reflecting similarity between the shapes, and the total elastic energy accounting for the growth of the tissue. We apply the proposed shape registration framework to study zebrafish embryo epiboly process and tissue expansion during skin reconstruction surgery. We anticipate that our PDE-constrained shape registration method will improve our understanding of biological and medical problems in which tissues undergo extreme deformations over time.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge