Reduced Overhead Channel Estimation for OTFS With Split Pilot

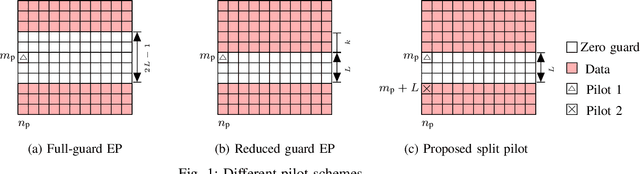
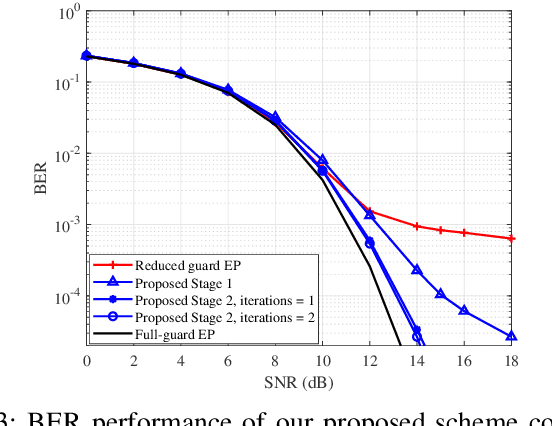
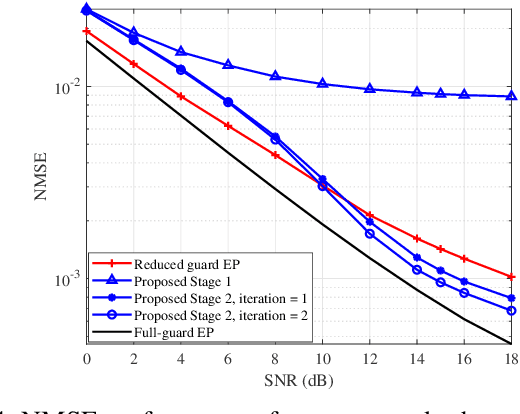
Orthogonal time frequency space modulation (OTFS) is currently one of the most robust modulation techniques for high Doppler channels. However, to reap the benefits of OTFS, an accurate channel estimation is crucial. To this mean, the widely used embedded pilot structures use twice the channel length size as a delay guard to avoid interference between the pilot and data symbols. Hence, incurring a large spectral efficiency loss, especially in wideband systems where the channel length is large. To reduce the pilot overhead, we propose a novel split pilot structure with two impulse pilots. With two pilots, we can use one to cancel the other, thus, capable of removing the pilot interference over data. To remove the data interference from the pilot, we also propose an iterative joint channel estimation and detection technique tailored to the proposed split pilot structure. With the interference caused by the delay spread solved, we reduce the number of delay guards in our system by half, significantly improving the spectral efficiency. To corroborate our claims, we numerically demonstrate that our proposed method can achieve performance levels comparable to that of the full-guard method while using only half the delay guard. Additionally, we show that our proposed iterative channel estimating technique has a fast convergence speed, requiring only two iterations.

Joint Channel Estimation and Equalization in Massive MIMO Using a Single Pilot Subcarrier

The focus of this letter is on the reduction of the large pilot overhead in orthogonal frequency division multiplexing (OFDM) based massive multiple-input multiple-output (MIMO) systems. We propose a novel joint channel estimation and equalization technique that requires only one pilot subcarrier, reducing the pilot overhead by orders of magnitude. We take advantage of the coherent bandwidth spanning over multiple subcarrier bands. This allows for a band of subcarriers to be equalized with the channel frequency response (CFR) at a single subcarrier. Subsequently, the detected data symbols are considered as virtual pilots, and their CFRs are updated without additional pilot overhead. Thereafter, the remaining channel estimation and equalization can be performed in a sliding manner. With this approach, we use multiple channel estimates to equalize the data at each subcarrier. This allows us to take advantage of frequency diversity and improve the detection performance. Finally, we corroborate the above claims through extensive numerical analysis, showing the superior performance of our proposed technique compared to conventional methods.

OTFS Without CP in Massive MIMO: Breaking Doppler Limitations with TR-MRC and Windowing

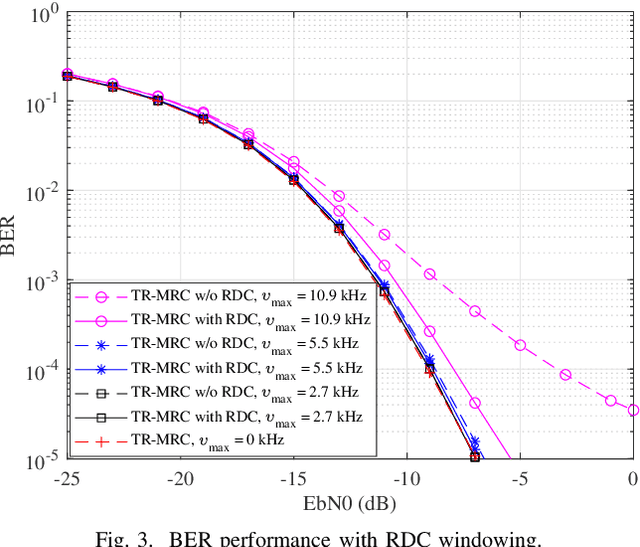
Orthogonal time frequency space (OTFS) modulation has recently emerged as an effective waveform to tackle the linear time-varying channels. In OTFS literature, approximately constant channel gains for every group of samples within each OTFS block are assumed. This leads to limitations for OTFS on the maximum Doppler frequency that it can tolerate. Additionally, presence of cyclic prefix (CP) in OTFS signal limits the flexibility in adjusting its parameters to improve its robustness against channel time variations. Therefore, in this paper, we study the possibility of removing the CP overhead from OTFS and breaking its Doppler limitations through multiple antenna processing in the large antenna regime. We asymptotically analyze the performance of time-reversal maximum ratio combining (TR-MRC) for OTFS without CP. We show that doubly dispersive channel effects average out in the large antenna regime when the maximum Doppler shift is within OTFS limitations. However, for considerably large Doppler shifts exceeding OTFS limitations, a residual Doppler effect remains. Our asymptotic derivations reveal that this effect converges to scaling of the received symbols in delay dimension with the samples of a Bessel function that depends on the maximum Doppler shift. Hence, we propose a novel residual Doppler correction (RDC) windowing technique that can break the Doppler limitations of OTFS and lead to a performance close to that of the linear time-invariant channels. Finally, we confirm the validity of our claims through simulations.