Modeling Magnetic Particle Imaging for Dynamic Tracer Distributions

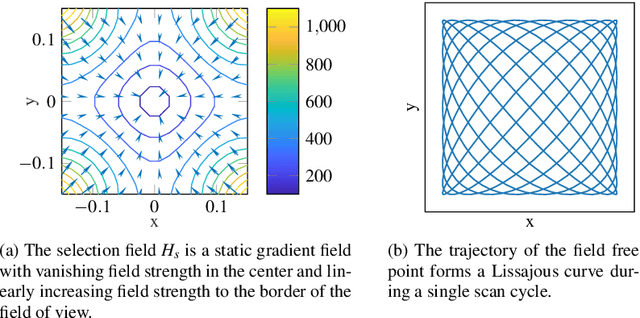
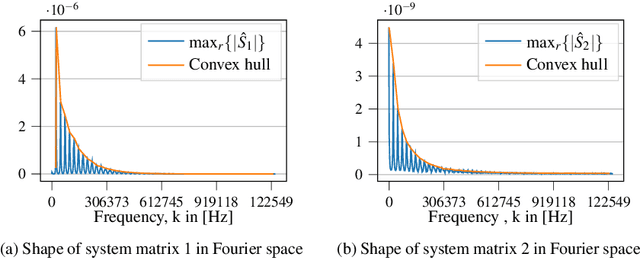
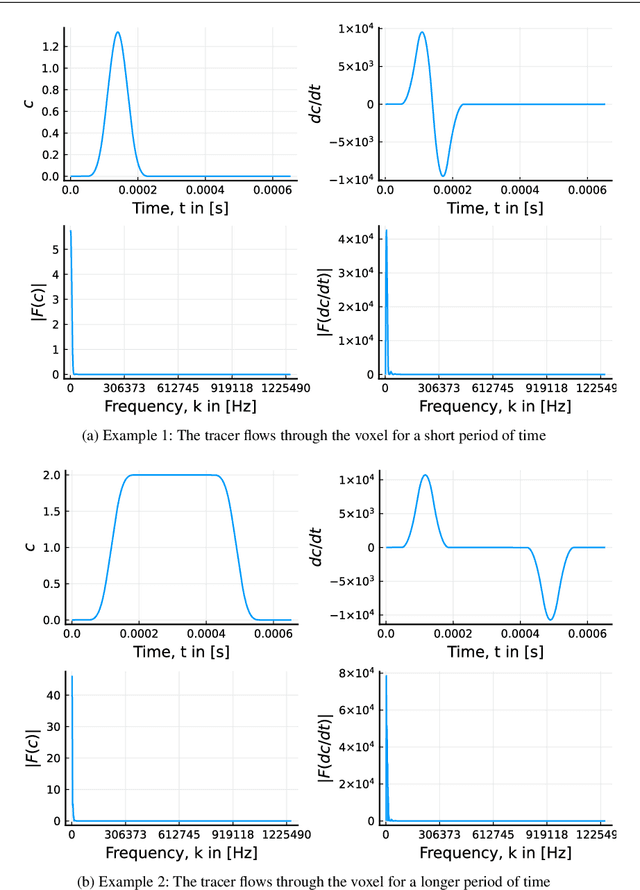
Magnetic Particle Imaging (MPI) is a promising tracer-based, functional medical imaging technique which measures the non-linear response of superparamagnetic iron oxide nanoparticles (SPION) to a dynamic magnetic field. For image reconstruction, system matrices from time-consuming calibration scans are used predominantly. Finding modeled forward operators for magnetic particle imaging, which are able to compete with measured matrices in practice, is an ongoing topic of research. The existing models for magnetic particle imaging are by design not suitable for dynamic tracer concentrations. Neither modeled nor measured system matrices account for changes in the concentration during a single scanning cycle. In this paper we present a new MPI forward model for dynamic concentrations. A standard model will be introduced briefly, followed by the changes due to the dynamic behavior of the tracer concentration. Furthermore, the relevance of this new extended model is examined by investigating the influence of the extension and example reconstructions with the new and the standard model.

Polygon Exploration with Time-Discrete Vision

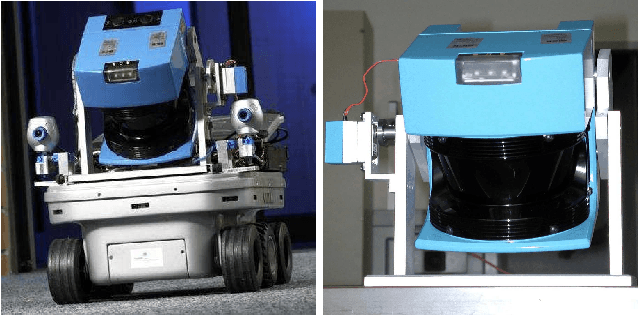
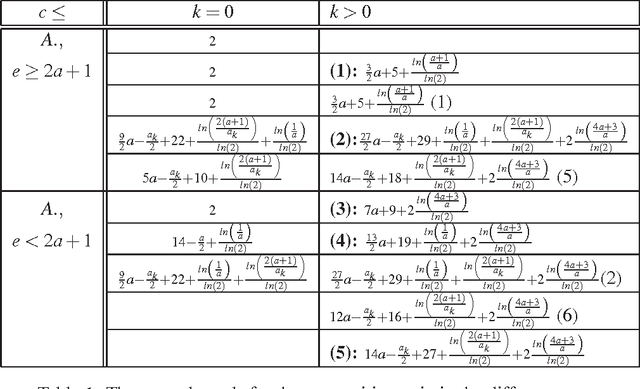
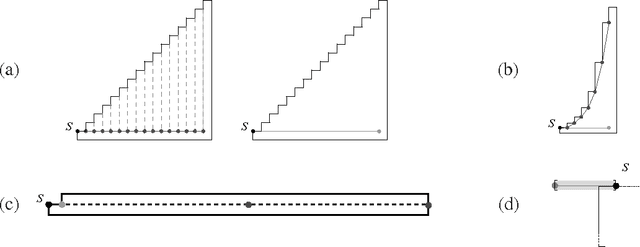
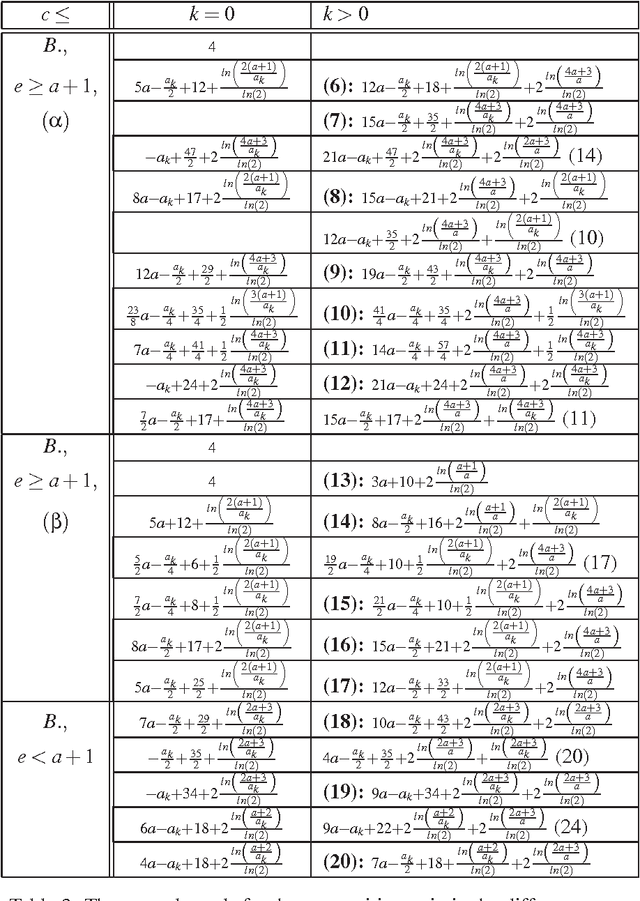
With the advent of autonomous robots with two- and three-dimensional scanning capabilities, classical visibility-based exploration methods from computational geometry have gained in practical importance. However, real-life laser scanning of useful accuracy does not allow the robot to scan continuously while in motion; instead, it has to stop each time it surveys its environment. This requirement was studied by Fekete, Klein and Nuechter for the subproblem of looking around a corner, but until now has not been considered in an online setting for whole polygonal regions. We give the first algorithmic results for this important algorithmic problem that combines stationary art gallery-type aspects with watchman-type issues in an online scenario: We demonstrate that even for orthoconvex polygons, a competitive strategy can be achieved only for limited aspect ratio A (the ratio of the maximum and minimum edge length of the polygon), i.e., for a given lower bound on the size of an edge; we give a matching upper bound by providing an O(log A)-competitive strategy for simple rectilinear polygons, using the assumption that each edge of the polygon has to be fully visible from some scan point.