A Theory of Learnability for Offline Decision Making

We study the problem of offline decision making, which focuses on learning decisions from datasets only partially correlated with the learning objective. While previous research has extensively studied specific offline decision making problems like offline reinforcement learning (RL) and off-policy evaluation (OPE), a unified framework and theory remain absent. To address this gap, we introduce a unified framework termed Decision Making with Offline Feedback (DMOF), which captures a wide range of offline decision making problems including offline RL, OPE, and offline partially observable Markov decision processes (POMDPs). For the DMOF framework, we introduce a hardness measure called the Offline Estimation Coefficient (OEC), which measures the learnability of offline decision making problems and is also reflected in the derived minimax lower bounds. Additionally, we introduce an algorithm called Empirical Decision with Divergence (EDD), for which we establish both an instance-dependent upper bound and a minimax upper bound. The minimax upper bound almost matches the lower bound determined by the OEC. Finally, we show that EDD achieves a fast convergence rate (i.e., a rate scaling as $1/N$, where $N$ is the sample size) for specific settings such as supervised learning and Markovian sequential problems~(e.g., MDPs) with partial coverage.

Offline Reinforcement Learning with Additional Covering Distributions

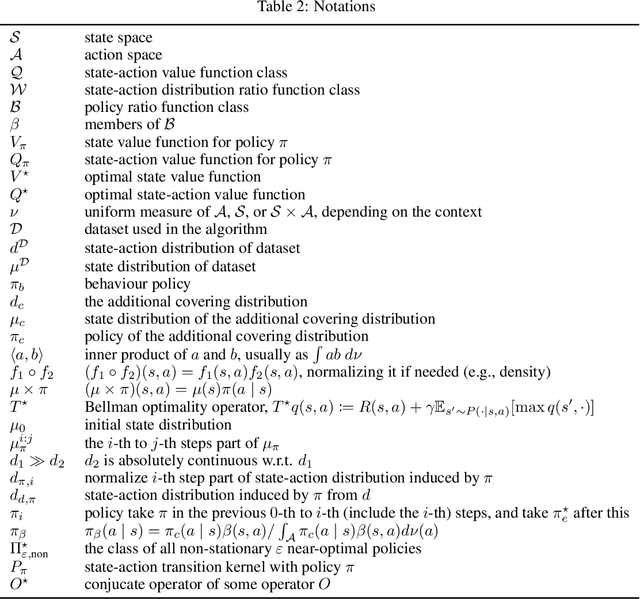
We study learning optimal policies from a logged dataset, i.e., offline RL, with function approximation. Despite the efforts devoted, existing algorithms with theoretic finite-sample guarantees typically assume exploratory data coverage or strong realizable function classes, which is hard to be satisfied in reality. While there are recent works that successfully tackle these strong assumptions, they either require the gap assumptions that only could be satisfied by part of MDPs or use the behavior regularization that makes the optimality of learned policy even intractable. To solve this challenge, we provide finite-sample guarantees for a simple algorithm based on marginalized importance sampling (MIS), showing that sample-efficient offline RL for general MDPs is possible with only a partial coverage dataset and weak realizable function classes given additional side information of a covering distribution. Furthermore, we demonstrate that the covering distribution trades off prior knowledge of the optimal trajectories against the coverage requirement of the dataset, revealing the effect of this inductive bias in the learning processes.