Three-agent Time-constrained Cooperative Pursuit-Evasion
Paper and Code
Jun 03, 2021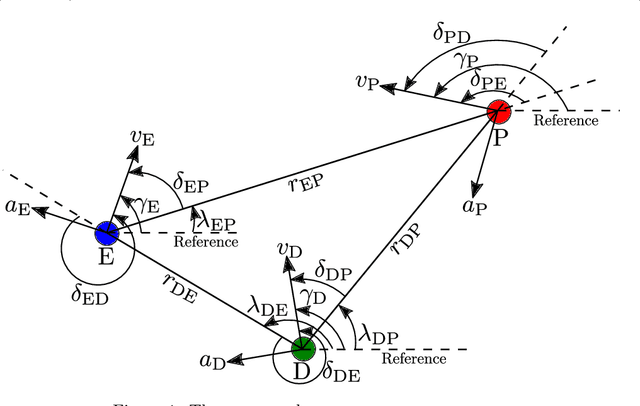
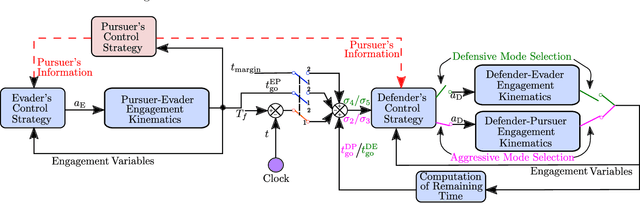
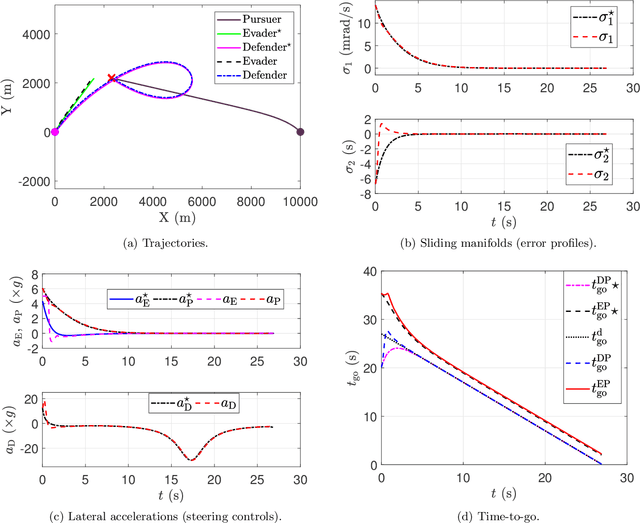
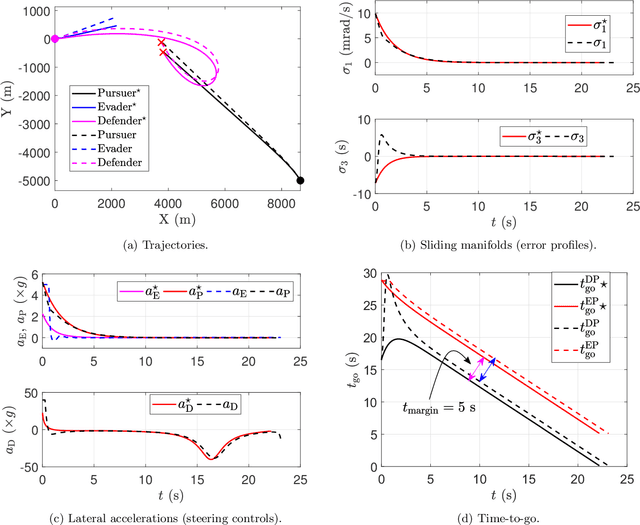
This paper considers a pursuit-evasion scenario among three agents -- an evader, a pursuer, and a defender. We design cooperative guidance laws for the evader and the defender team to safeguard the evader from an attacking pursuer. Unlike differential games, optimal control formulations, and other heuristic methods, we propose a novel perspective on designing effective nonlinear feedback control laws for the evader-defender team using a time-constrained guidance approach. The evader lures the pursuer on the collision course by offering itself as bait. At the same time, the defender protects the evader from the pursuer by exercising control over the engagement duration. Depending on the nature of the mission, the defender may choose to take an aggressive or defensive stance. Such consideration widens the applicability of the proposed methods in various three-agent motion planning scenarios such as aircraft defense, asset guarding, search and rescue, surveillance, and secure transportation. We use a fixed-time sliding mode control strategy to design the control laws for the evader-defender team and a nonlinear finite-time disturbance observer to estimate the pursuer's maneuver. Finally, we present simulations to demonstrate favorable performance under various engagement geometries, thus vindicating the efficacy of the proposed designs.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge