Smooth Deformation Field-based Mismatch Removal in Real-time
Paper and Code
Jul 16, 2020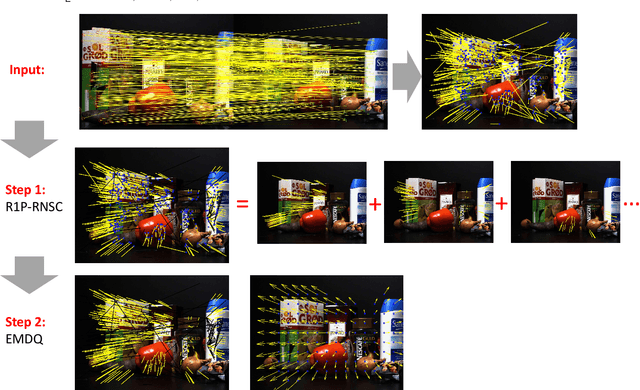


This paper studies the mismatch removal problem, which may serve as the subsequent step of feature matching. Non-rigid deformation makes it difficult to remove mismatches because no parametric transformation can be found. To solve this problem, we first propose an algorithm based on the re-weighting and 1-point RANSAC strategy (R1P-RNSC), which is a parametric method under a reasonable assumption that the non-rigid deformation can be approximately represented by multiple locally rigid transformations. R1P-RNSC is fast but suffers from a drawback that the local smoothing information cannot be taken into account. Then, we propose a non-parametric algorithm based on the expectation maximization algorithm and dual quaternion (EMDQ) representation to generate the smooth deformation field. The two algorithms compensate for the drawbacks of each other. Specifically, EMDQ needs good initial values provided by R1P-RNSC, and R1P-RNSC needs EMDQ for refinement. Experimental results with real-world data demonstrate that the combination of the two algorithms has the best accuracy compared to other state-of-the-art methods, which can handle up to 85% of outliers in real-time. The ability to generate dense deformation field from sparse matches with outliers in real-time makes the proposed algorithms have many potential applications, such as non-rigid registration and SLAM.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge