Relaxed Survey Propagation for The Weighted Maximum Satisfiability Problem
Paper and Code
Jan 15, 2014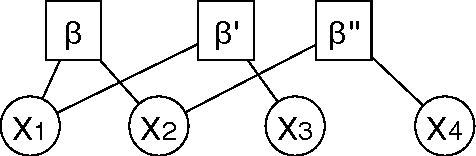
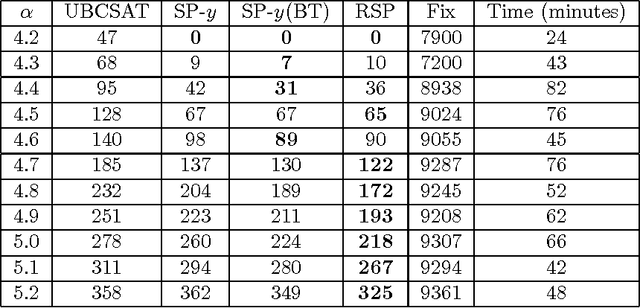
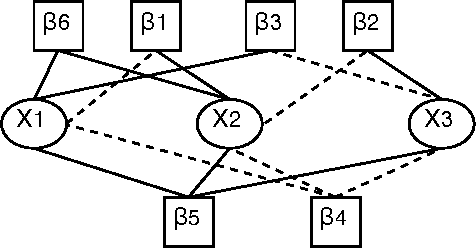
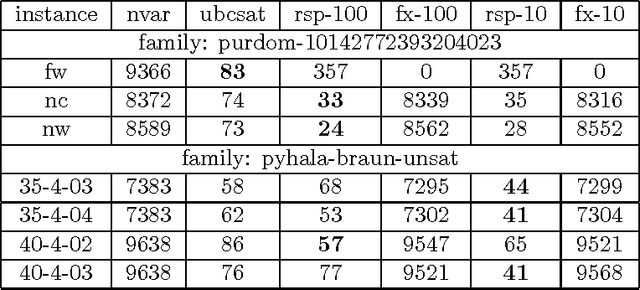
The survey propagation (SP) algorithm has been shown to work well on large instances of the random 3-SAT problem near its phase transition. It was shown that SP estimates marginals over covers that represent clusters of solutions. The SP-y algorithm generalizes SP to work on the maximum satisfiability (Max-SAT) problem, but the cover interpretation of SP does not generalize to SP-y. In this paper, we formulate the relaxed survey propagation (RSP) algorithm, which extends the SP algorithm to apply to the weighted Max-SAT problem. We show that RSP has an interpretation of estimating marginals over covers violating a set of clauses with minimal weight. This naturally generalizes the cover interpretation of SP. Empirically, we show that RSP outperforms SP-y and other state-of-the-art Max-SAT solvers on random Max-SAT instances. RSP also outperforms state-of-the-art weighted Max-SAT solvers on random weighted Max-SAT instances.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge