Re-ranking Based Diversification: A Unifying View
Paper and Code
Jun 26, 2019
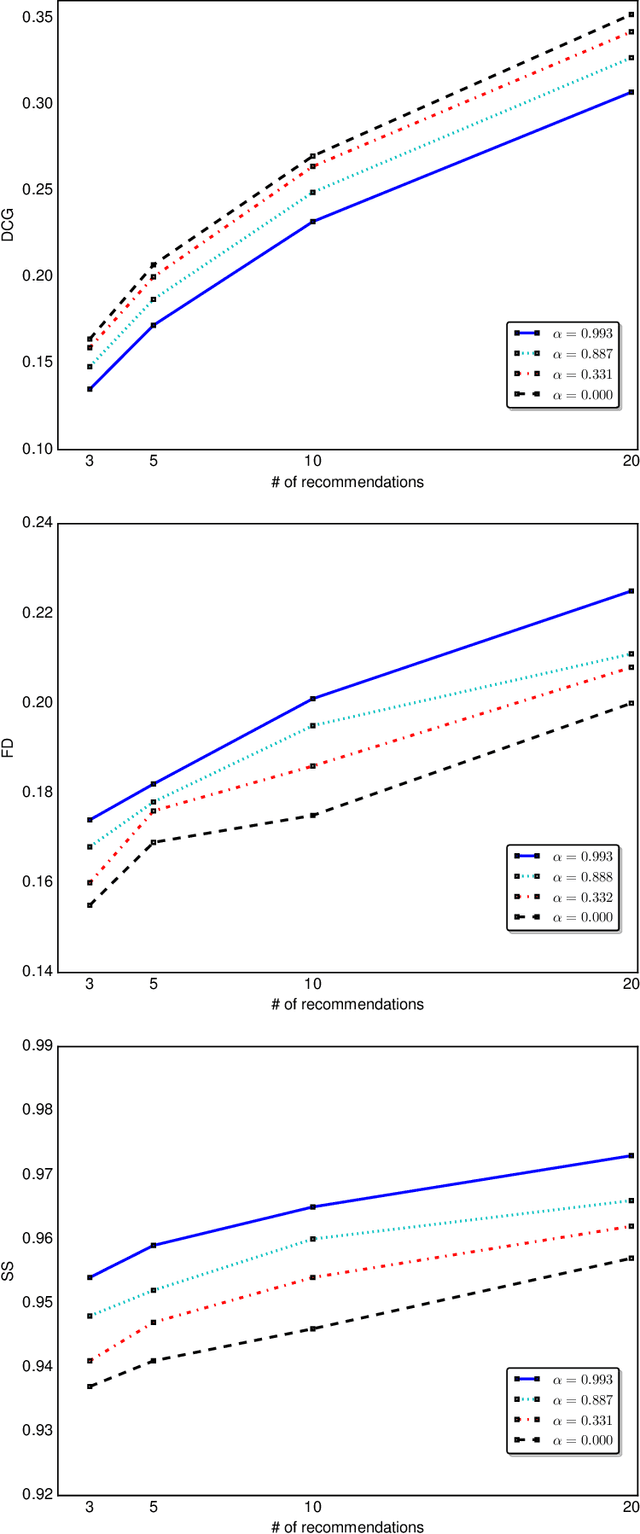
We analyze different re-ranking algorithms for diversification and show that majority of them are based on maximizing submodular/modular functions from the class of parameterized concave/linear over modular functions. We study the optimality of such algorithms in terms of the `total curvature'. We also show that by adjusting the hyperparameter of the concave/linear composition to trade-off relevance and diversity, if any, one is in fact tuning the `total curvature' of the function for relevance-diversity trade-off.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge