Radar Odometry on SE(3) with Constant Acceleration Motion Prior and Polar Measurement Model
Paper and Code
Sep 11, 2022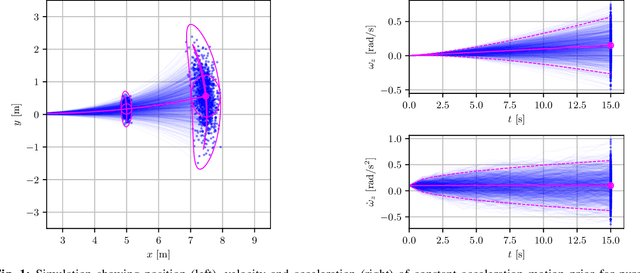
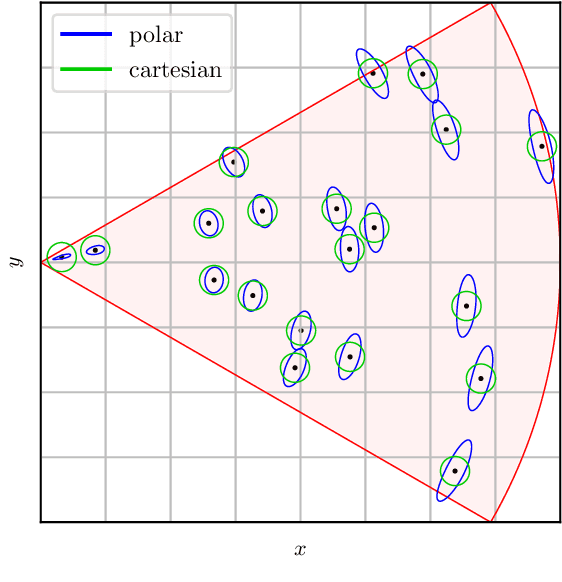
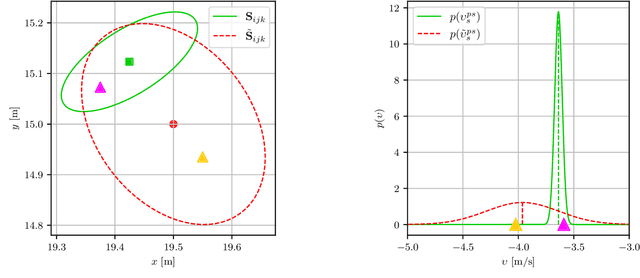
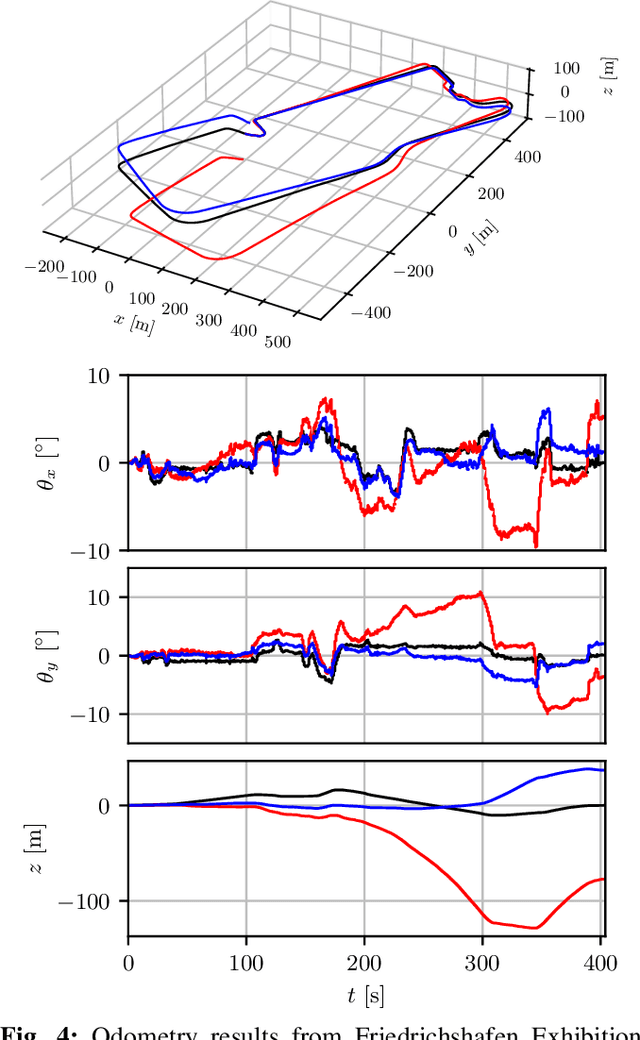
This paper presents an approach to radar odometry on $SE(3)$ which utilizes a constant acceleration motion prior. The motion prior is integrated into a sliding window optimization scheme. We use the Magnus expansion to accurately integrate the motion prior while maintaining real-time performance. In addition, we adopt a polar measurement model to better represent radar detection uncertainties. Our estimator is evaluated using a large real-world dataset from a prototype high-resolution radar sensor. The new motion prior and measurement model signifcantly improve odometry performance relative to the constant velocity motion prior and Cartesian measurement model from our previous work, particularly in roll, pitch and height.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge