PSLF: A PID Controller-incorporated Second-order Latent Factor Analysis Model for Recommender System
Paper and Code
Aug 31, 2024


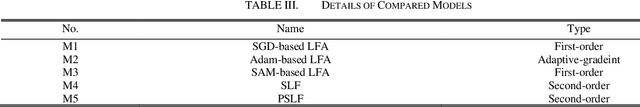
A second-order-based latent factor (SLF) analysis model demonstrates superior performance in graph representation learning, particularly for high-dimensional and incomplete (HDI) interaction data, by incorporating the curvature information of the loss landscape. However, its objective function is commonly bi-linear and non-convex, causing the SLF model to suffer from a low convergence rate. To address this issue, this paper proposes a PID controller-incorporated SLF (PSLF) model, leveraging two key strategies: a) refining learning error estimation by incorporating the PID controller principles, and b) acquiring second-order information insights through Hessian-vector products. Experimental results on multiple HDI datasets indicate that the proposed PSLF model outperforms four state-of-the-art latent factor models based on advanced optimizers regarding convergence rates and generalization performance.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge