Pattern Formation for Asynchronous Robots without Agreement in Chirality
Paper and Code
Mar 11, 2014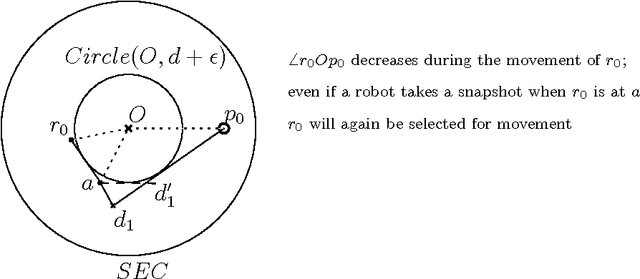
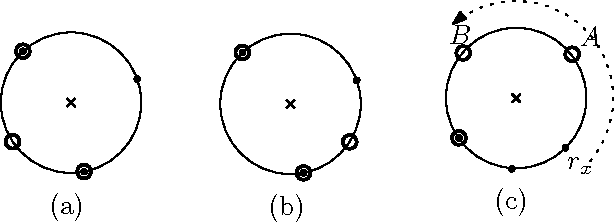
This paper presents a deterministic algorithm for forming a given asymmetric pattern in finite time by a set of autonomous, homogeneous, oblivious mobile robots under the CORDA model. The robots are represented as points on the 2D plane. There is no explicit communication between the robots. The robots coordinate among themselves by observing the positions of the other robots on the plane. Initially all the robots are assumed to be stationary. The robots have local coordinate systems defined by Sense of Direction (SoD), orientation or chirality and scale. Initially the robots are in asymmetric configuration. We show that these robots can form any given asymmetric pattern in finite time.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge