Output Mode Switching for Parallel Five-bar Manipulators Using a Graph-based Path Planner
Paper and Code
Sep 22, 2022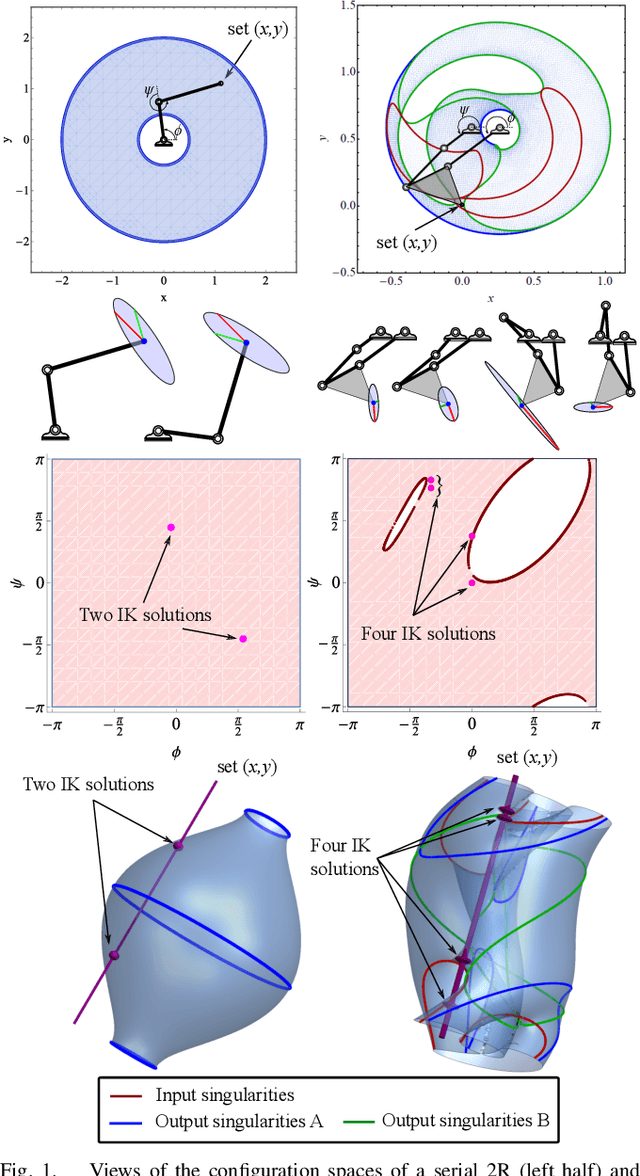
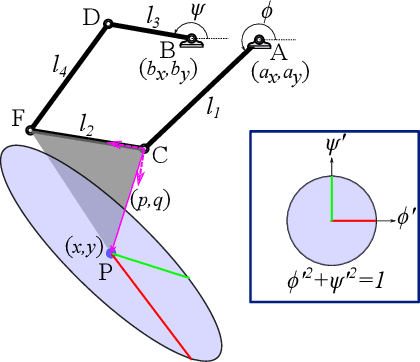

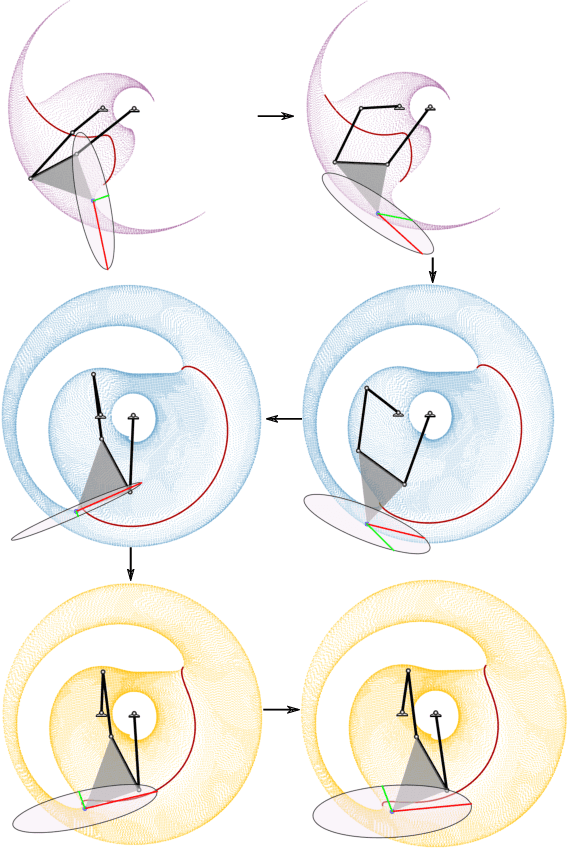
The configuration manifolds of parallel manipulators exhibit more nonlinearity than serial manipulators. Qualitatively, they can be seen to possess extra folds. By projecting such manifolds onto spaces of engineering relevance, such as an output workspace or an input actuator space, these folds cast edges that exhibit nonsmooth behavior. For example, inside the global workspace bounds of a five-bar linkage appear several local workspace bounds that only constrain certain output modes of the mechanism. The presence of such boundaries, which manifest in both input and output projections, serve as a source of confusion when these projections are studied exclusively instead of the configuration manifold itself. Particularly, the design of nonsymmetric parallel manipulators has been confounded by the presence of exotic projections in their input and output spaces. In this paper, we represent the configuration space with a radius graph, then weight each edge by solving an optimization problem using homotopy continuation to quantify transmission quality. We then employ a graph path planner to approximate geodesics between configuration points that avoid regions of low transmission quality. Our methodology automatically generates paths capable of transitioning between non-neighboring output modes, a motion which involves osculating multiple workspace boundaries (local, global, or both). We apply our technique to two nonsymmetric five-bar examples that demonstrate how transmission properties and other characteristics of the workspace can be selected by switching output modes.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge