Nash Neural Networks : Inferring Utilities from Optimal Behaviour
Paper and Code
Mar 25, 2022
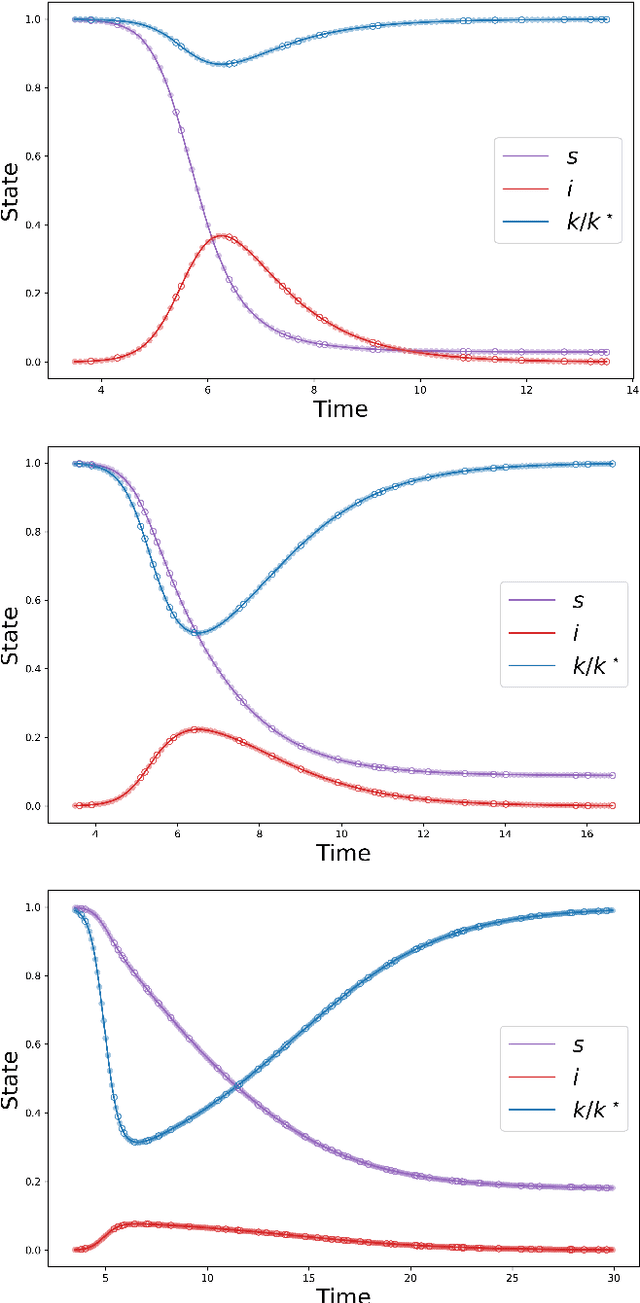
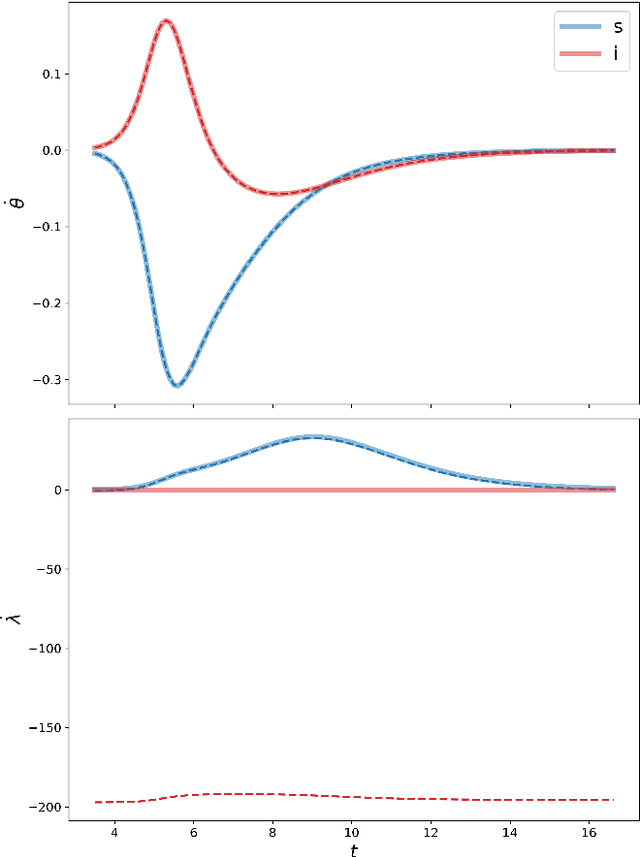

We propose Nash Neural Networks ($N^3$) as a new type of Physics Informed Neural Network that is able to infer the underlying utility from observations of how rational individuals behave in a differential game with a Nash equilibrium. We assume that the dynamics for both the population and the individual are known, but not the payoff function, which specifies the cost per unit time of being in any particular state. We construct our network in such a way that the Euler-Lagrange equations of the corresponding optimal control problem are satisfied and the optimal control is self-consistently determined. In this way, we are able to learn the unknown payoff function in an unsupervised manner. We have applied the $N^3$ to study the optimal behaviour during epidemics, in which individuals can choose to socially distance depending on the state of the pandemic and the cost of being infected. Training our network against synthetic data for a simple SIR model, we showed that it is possible to accurately reproduce the hidden payoff function, in such a way that the game dynamics are respected. Our approach will have far-reaching applications, as it allows one to infer utilities from behavioural data, and can thus be applied to study a wide array of problems in science, engineering, economics and government planning.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge